A parabola passes through the points (0,0) and (6,0) it the turning point of the parabola is T(h, 4), which statement must be true?About Press Copyright Contact us Creators Advertise Developers Terms Privacy Policy & Safety How works Test new features Press Copyright Contact us Creators A tutorial on how to complete the square and how we can use this new form to find the turning point of a parabola
2
How to find the turning point of a parabola from an equation
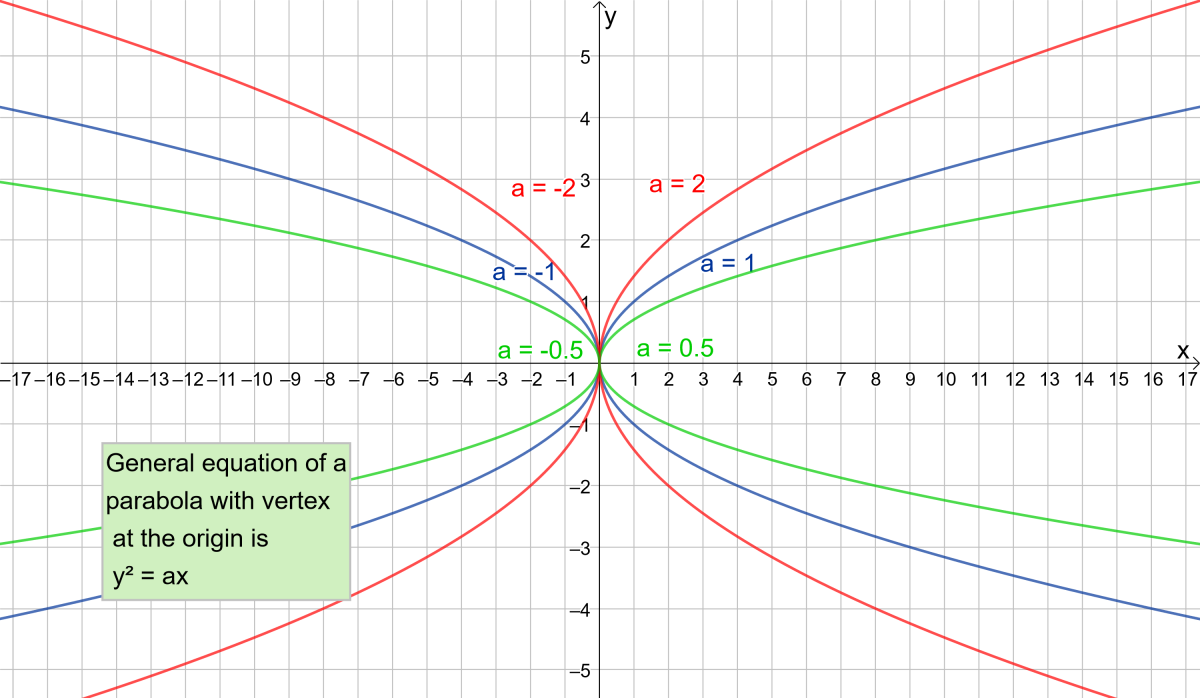
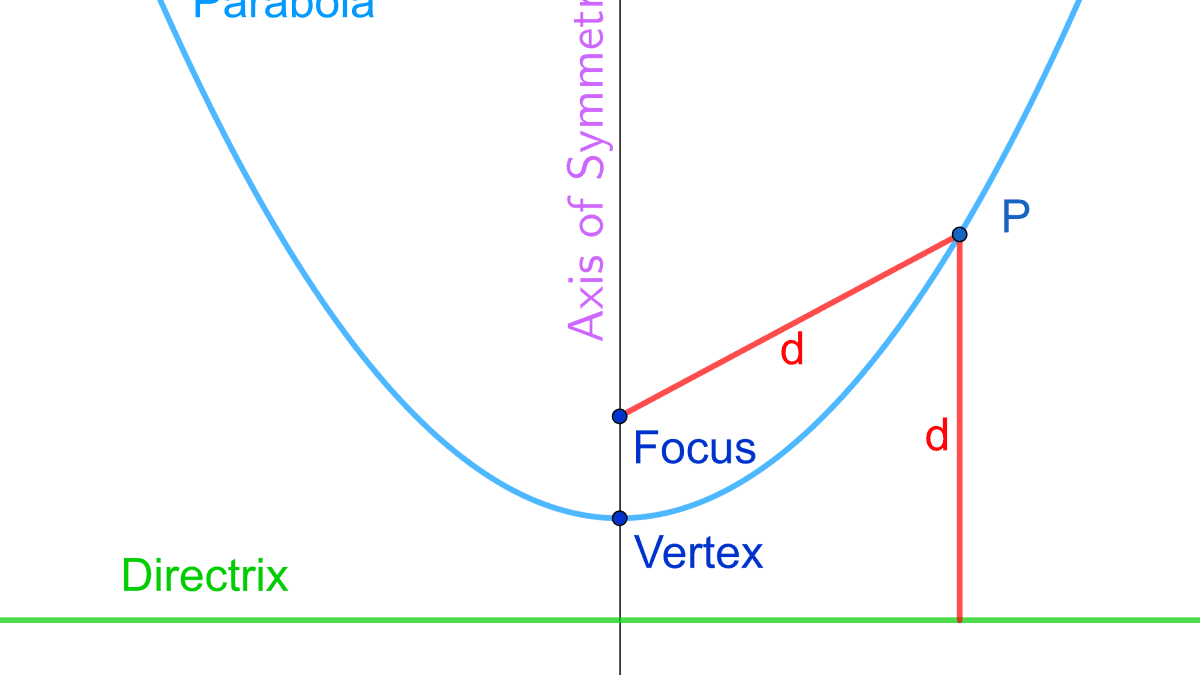
How to find the turning point of a parabola from an equation-A second approach is to find the turning point of the parabola Completing the square, we have \\begin{align*} y &= x^2 2ax 1 \\ &= (x a)^2 1 a^2, \end{align*}\ so the minimum occurs when \(x = a\) and then \(y = 1 a^2\) So the turning point is at \(a, 1 a^2)\The parabola is the locus of points in that plane that are equidistant from both the directrix and the focus Another description of a parabola is as a conic section, created from the intersection of a right circular conical surface and a plane parallel to another plane that



Parabola Equations And Graphs Directrix And Focus And How To Find Roots Of Quadratic Equations Owlcation
How to find the turning point (vertex) of a quadratic curve, equation or graph Read more xenon lights Follow 0 Comments – 12 12 – 24 – 12 = 24 this is the ycoordinate of the vertex So the vertex (turning point of this parabola is (2,24) HOW TO CALCULATE THE VERTEX (TURNING POINT) Recommended Explore professional development books with Scribd ScribdThe point (or points) where the parabola crosses the xaxis Also known as the roots, solutions, or zeros yintercept The point where the parabola crosses the yaxis Maximum The highest point on a parabola Minimum The lowest point on a parabola What do the turning point and the line of symmetry have in common The equation for the line of symmetry is x= the x value of the turning pointFinding the turning point and the line of symmetry Higher The coordinates of the turning point and the equation of the line of symmetry can be found by writing the quadratic expression in
A turning point may be either a local maximum or a minimum point If the function is smooth, then the turning point must be a stationary point, however not all stationary points are turning points, for example has a stationary point at x=0, but the derivative doesn't change sign as there is a point of inflexion at x=0 Curve sketching The given x intercept (x1,0) satisfies this equation, so 0 = a(x1 −h)2 k and hence a = − k (x1 − h)2 Note that this is undefined in the case where x1 = h That is, when the vertex is an x intercept, resulting in an indeterminate value for a (any value would result in a parabolaThe vertex of a parabola is the highest or lowest point, also known as the maximum or minimum of a parabola Properties of the Vertex of a Parabola is the maximum or minimum value of the parabola (see picture below) is the turning point of the parabola
(x1x2)/2 where x1 and x2 are the intercepts of a parabola function Type your answer here Why you think that the midpoint of the xintercepts is the xcoordinates ofTP?The point or points where the graph crosses the xaxis Xintercept The vertical line that goes through the xcoordinate of the vertex and divides the parabola into 2 halvesAbout Press Copyright Contact us Creators Advertise Developers Terms Privacy Policy & Safety How works Test new features Press Copyright Contact us Creators



Corbettmaths Com Wp Content Uploads 19 01 Solving Quadratics Graphically Pdf 1 Pdf



2
How you think you find the turning point given the xintercepts of a parabola?To find what type of turningA parabola is defined as a collection of points such that the distance to a fixed point (the focus) and a fixed straight line (the directrix) are equal But it's probably easier to remember it as the Ushaped curved line created when a quadratic is graphed Many realworld objects travel in a parabolic shape When you shoot a basketball, the path of the ball creates a parabola Example



Www Whiteplainspublicschools Org Cms Lib Ny Centricity Domain 360 parabolas and properties packet Pdf



Parabola Turning Point P23
The turning point of f(x) is above the x axis For q < 0, f(x) is shifted vertically downwards by q units The turning point of f(x) is below the x axis q is also the y intercept of the parabolaParabolas always have a lowest point (or a highest point, if the parabola is upsidedown) This point, where the parabola changes direction, is called the "vertex" If the quadratic is written in the form y =If the parabola opens down, the vertex represents the highest point on the graph, or the maximum value In either case, the vertex is a turning point on the graph The graph is also symmetric with a vertical line drawn through the vertex, called the axis of symmetry How do you find the turning point of a derivative?




Turning Points And Intercepts Of A Parabola Function Geogebra




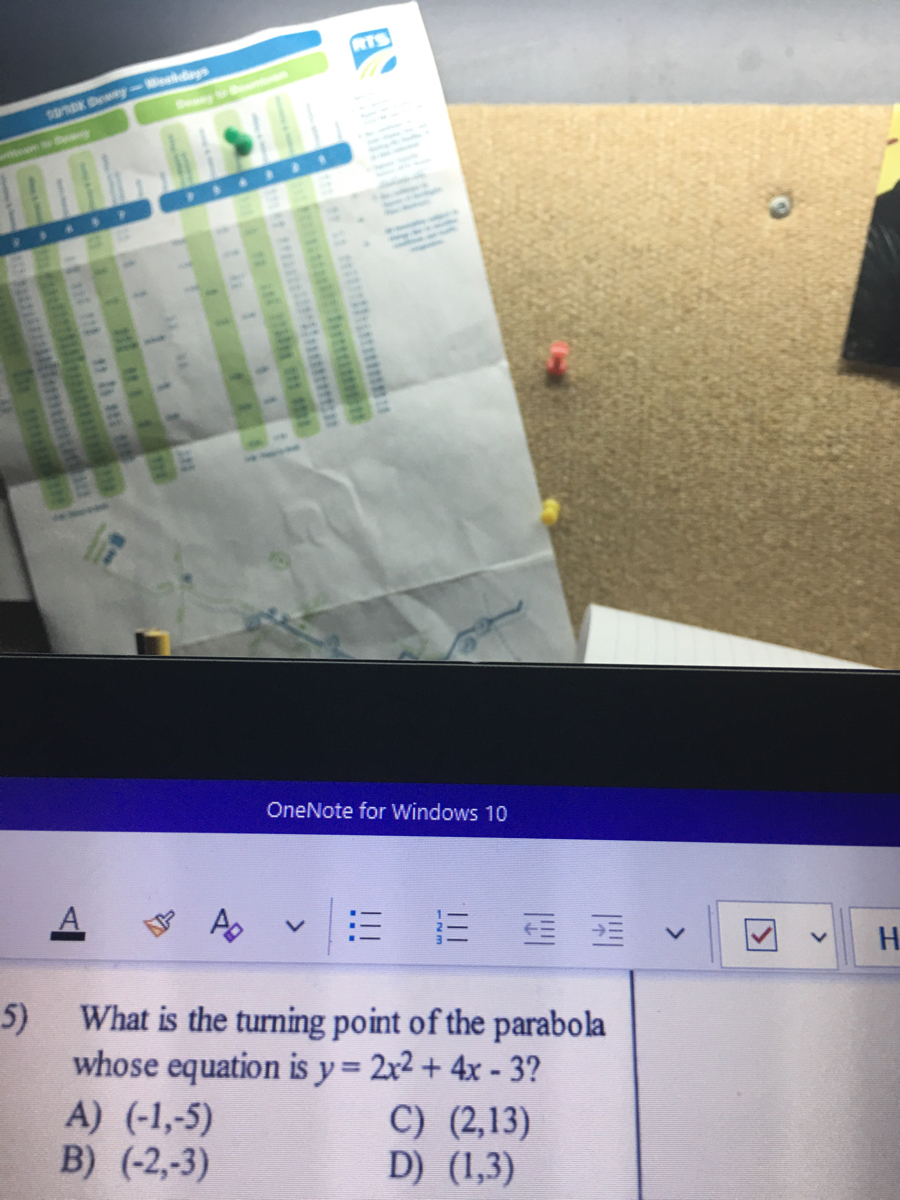
3 The Parabola Fx Has Turning Point A 3 2 And Poin Gauthmath
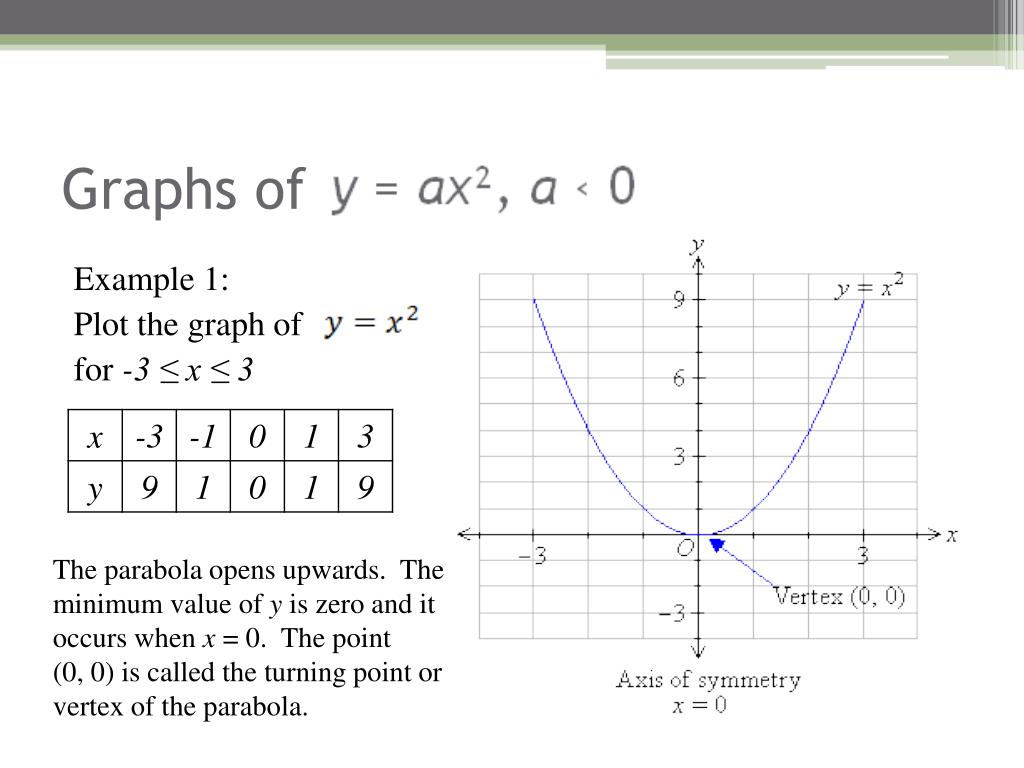
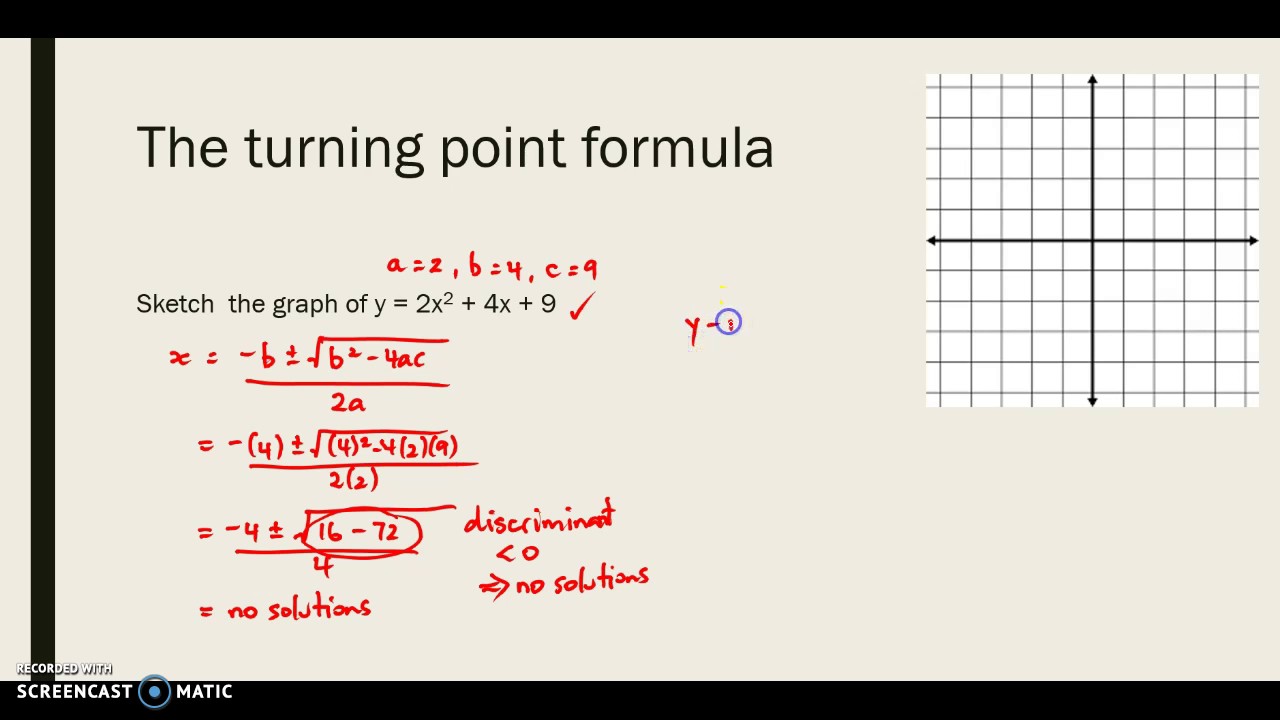
The parabola opens upwards The minimum value of y is zero and it occurs when x = 0 The point (0, 0) is called the turning point or vertex of the parabola In general Example 2 Solution When we plot these points and join them with a smooth curve, we obtain the graph shown above NoteHow do you find the turning point of a parabola?11 PARABOLA Writing in turning point form In this video I explain how to write the equation of a parabola, given in standard form, in turning point form, by completing the square



Solved Question 5 A F 6 0 The Sketch Shows A Circle A Parabola Which Is The Graph Of F And A Straight Line Which Is The Graph Of G The Parabol Course Hero



2
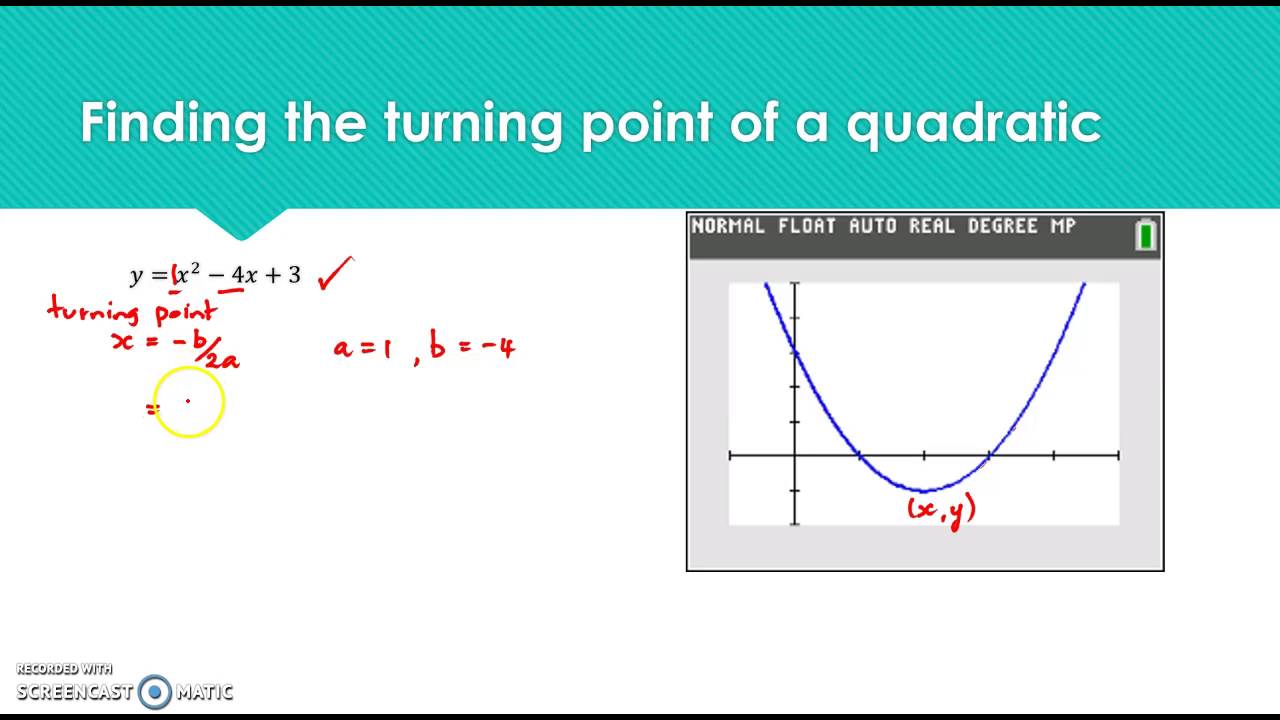
Show Answer The graph below shows a quadratic function with the following form y = a x 2 q Two points on the parabola are shown Point A, the turning point of the parabola, at ( 0;1 h = 2 11 If the parabola passes through (1, 2), then it must also pass through (5,2) 1 Point T is the highest point of the parabola y A II only B II only C I and II only D II and III only Answer To find the turning point of a parabola, first find it's xvalue, using the equation b/2a (from the quadratic form ax^2 bx c) For the given equation of parabola, you can find the vertex by completing the square in the form \ (\displaystyle y = a (xh)^2k\) where (h, k) is vertex The turning point is when the rate of change is zero



Ppt Quadratic Functions Powerpoint Presentation Free Download Id



Quadratic Functions Radical Quadratic And Rational Functions Idiot S Guides Algebra I
Given that the turning point of this parabola is (2,4) and 1 of the roots is (1,0), please find the equation of this parabola I started off by substituting the given numbers into the turning point form $0=a(x2)^24$ but i do not know where to put the roots in and form an equationPlease help thank youThe turning point of a parabola, also called the vertex, is the point on the parabola where the graph changes from increasing to decreasing, or fromThe parabola is the locus of points in that plane that are equidistant from both the directrix and the focus Another description of a parabola is as a conic section, created from the intersection of a right circular conical surface and a plane parallel to another plane that



Finding The Turning Point Of A Quadratic Graph Youtube




Quadratic Graphs Exercise Pdf Algebra Geometry
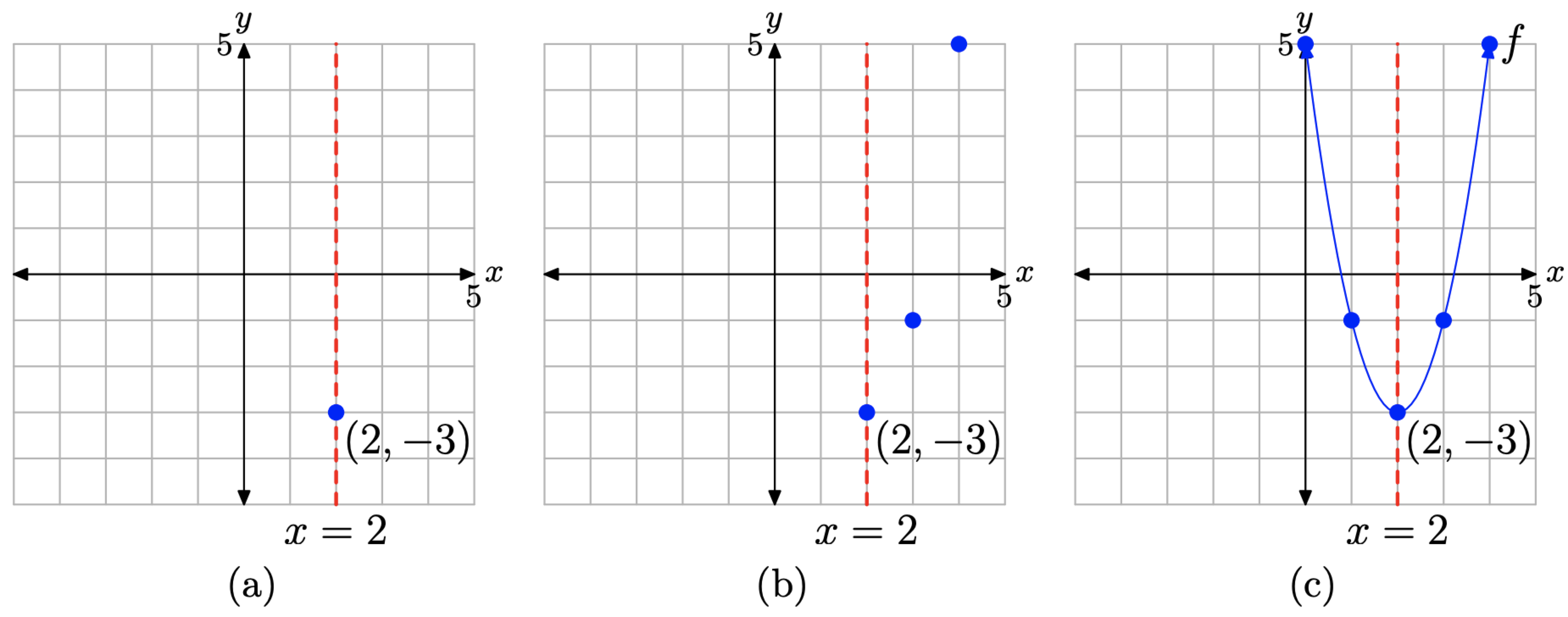
The parabola shown has a minimum turning point at (3, 2) The equation of the axis of symmetry is \ (x = 3\)How do you find the turning point of a parabola? After getting that, we are ready to conclude that the turning point of the parabola is exactly at 15,025 New questions in Math Coptodon zillii (gervais, 1848) redbelly tilapia The mathematics teacher will choose two students from a set of 6 equally qualified students 4 boys and 2 girls,to represent the class in the math comp etition Let A,B,C, and D represent the four



Www Lyonscsd Org Site Handlers Filedownload Ashx Moduleinstanceid 257 Dataid 2215 Filename 6 1 notes Pdf




Parabola Equations And Graphs Directrix And Focus And How To Find Roots Of Quadratic Equations Owlcation
5) Calculate the values of a and q The value of q is 3 The turning point of a parabola is the vertex; A Turning Point is a critical time in your life where big decisions could lead to big change, both in work and in life A Turning Point typically shows up about every 10 years of adult life between ages 18 and 65, but, of course, some experience fewer or more Turning Points and experience them at different times




Identifying Roots And Turning Points Of Quadratic Functions Gcse Mathematics Edexcel Revision Study Rocket
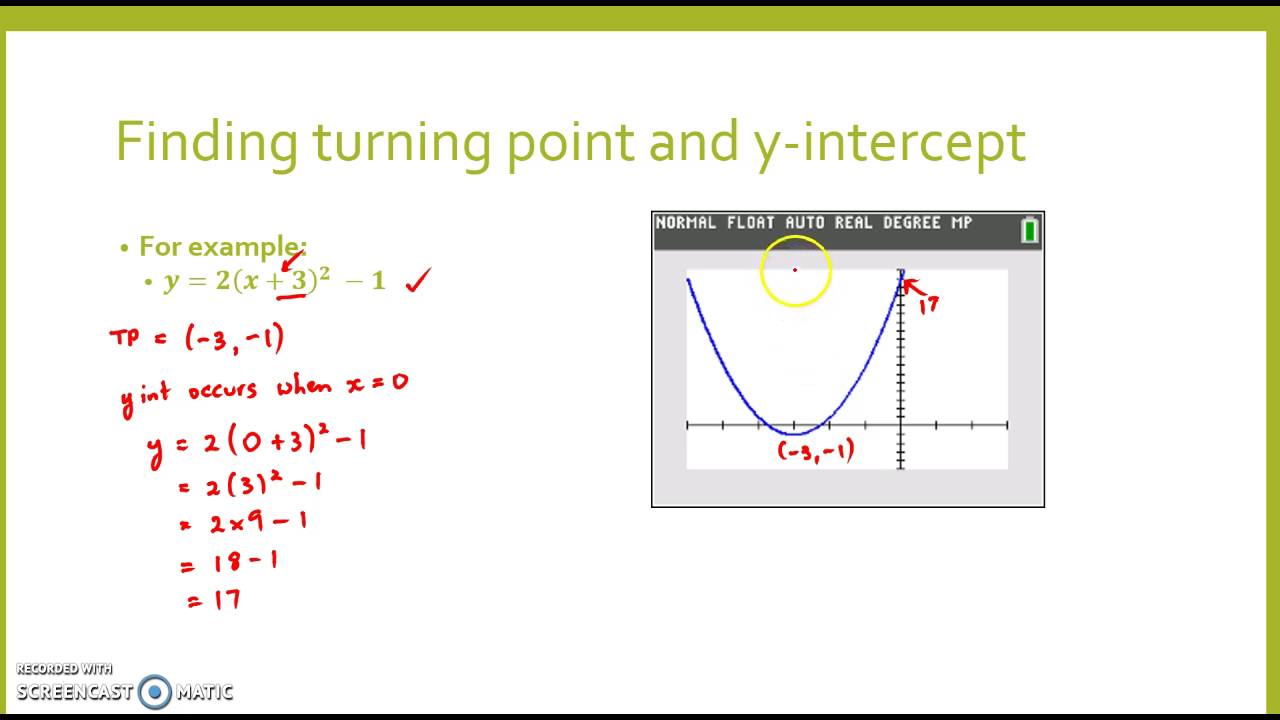



Finding Turning Point And Y Intercept Of Quadratic Equations Youtube
The turning point form of the formula is also the velocity equation The vertex is the peak of the parabola where the velocity, or rate of change, is zero How to find the turning point of a parabola The turning point, or the vertex can be found easily by differentiation The turning point is when the rate of change is zero You therefore differentiate f(x) and equate it to zero as shownTurning Points of Quadratic Graphs Graphs of quadratic functions have a vertical line of symmetry that goes through their turning pointThis means that the turning point is located exactly half way between the xaxis intercepts (if there are any!) There are two methods to find the turning point, Through factorising and completing the square Make sure you are happy with the following topics parabola passes through $4$ points 1 The locus of the midpoint of the line joining the focus to a moving point on another point on the parabola $y^2=4ax$ is another parabola




Sketching Quadratic Graphs Using The Turning Point Method Part 1 Ex 9 5 Youtube
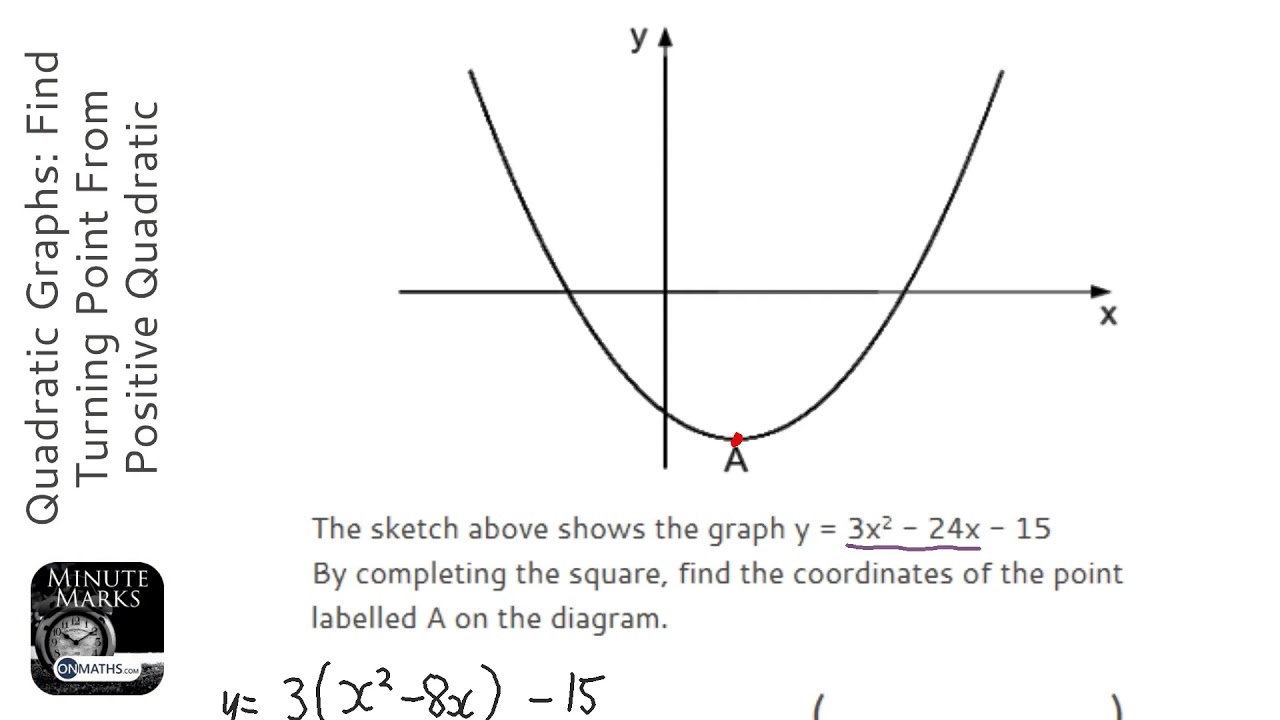



Quadratic Graphs Find Turning Point From Positive Quadratic Agt1 Grade 9 Gcse Maths Revision Youtube
To find the turning point of a parabola, first find it's xvalue, using the equation b/2a (from the quadratic form ax^2 bx c) 3 GeoGebra can be used very easily to find the equation of a parabola given three points, A, B, C input the command FitPoly{A, B, C}, 2 So, your new equation is y = 0^2 12 Now use algebra If, suppose equation of a parabola is \(\displaystyle yAuthor ithomson A quadratic function can be written in turning point form where Transformations of the graph of the quadratic can be explored by changing values of a, h and k 1 Use the slider to change the values of a Describe what happensIf the parabola opens down, the vertex represents the highest point on the graph, or the maximum value In either case, the vertex is a turning point on the graph The graph is also symmetric with a vertical line drawn through the vertex, called the axis of symmetry The y y intercept is the point at which the parabola crosses the y y axis




Lesson Explainer Graphing Quadratic Functions Nagwa
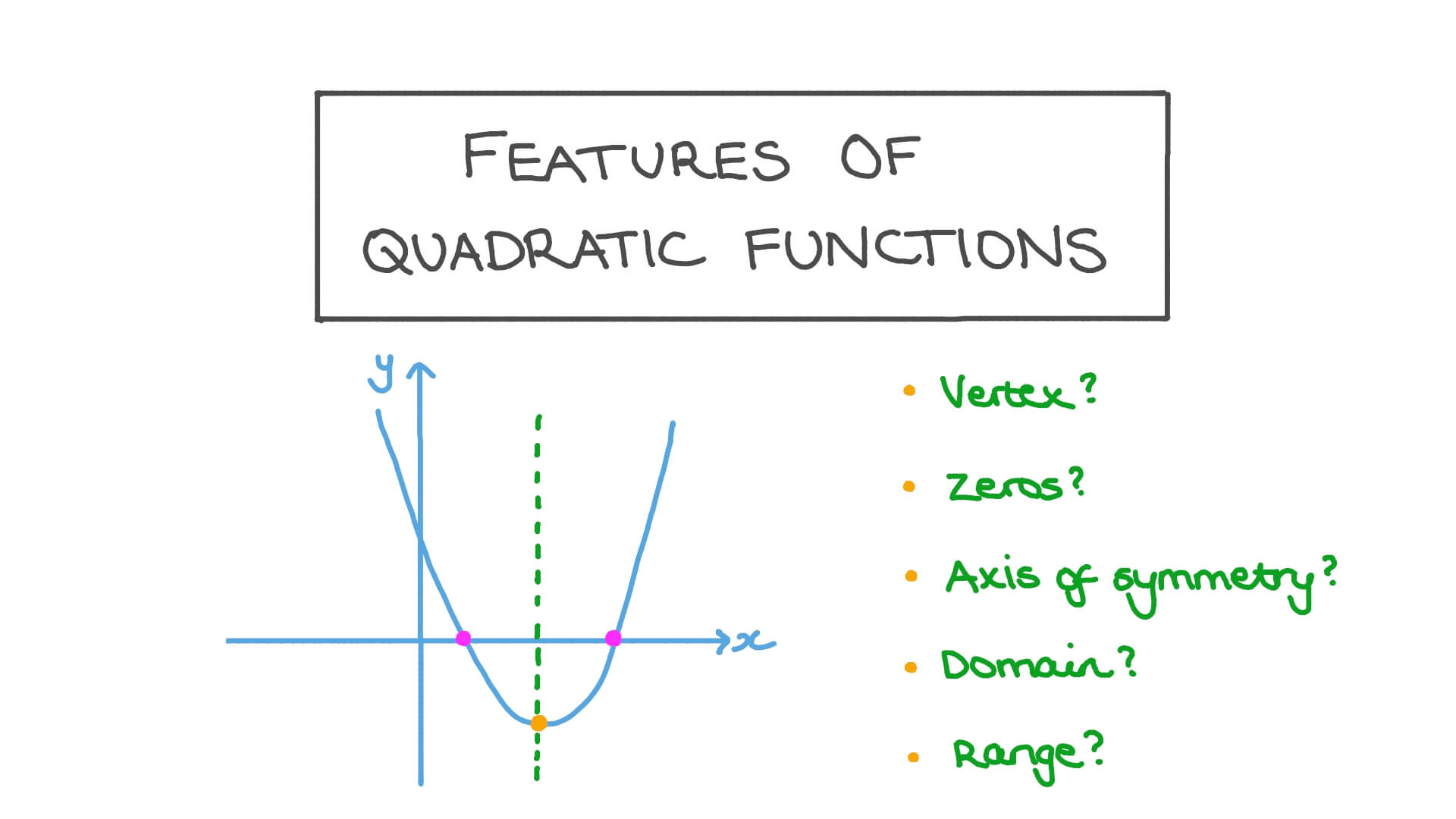



Lesson Video Features Of Quadratic Functions Nagwa
The turning Of the parabola is l) The parabola has a minimum point The parabola has two xintercepts 2r2 — Which point is on the graph of the parabola whose equation isThe Turning Point of a Parabola Is called the Vertex It is the Point at which the Value is Maximum If you really think I'm right, please UPVOTEThe Ushaped graph of a quadratic equation in the form of y = ax2 bx c is called a parabola The roots of the equation are the point (s) where the parabola crosses the xaxis These are the solutions found by factorizing or by using the quadratic formula There may be two, one or no roots The turning point is the point where the graph turns



Www Whiteplainspublicschools Org Cms Lib Ny Centricity Domain 360 parabolas and properties packet Pdf



2
− 3), and Point B is at ( 2; The turning point of a graph is where the curve in the graph turns The turning point will always be the minimum or the maximum value of your graph The parabola ( the curve) is symmetrical If we know the x value we can work out the y value!This is also it's highest or lowest point The graph below has a turning point (3, 2) xintercepts in greater depth now find your y value by using the x value you just found by plugging it into your function In example 3



2
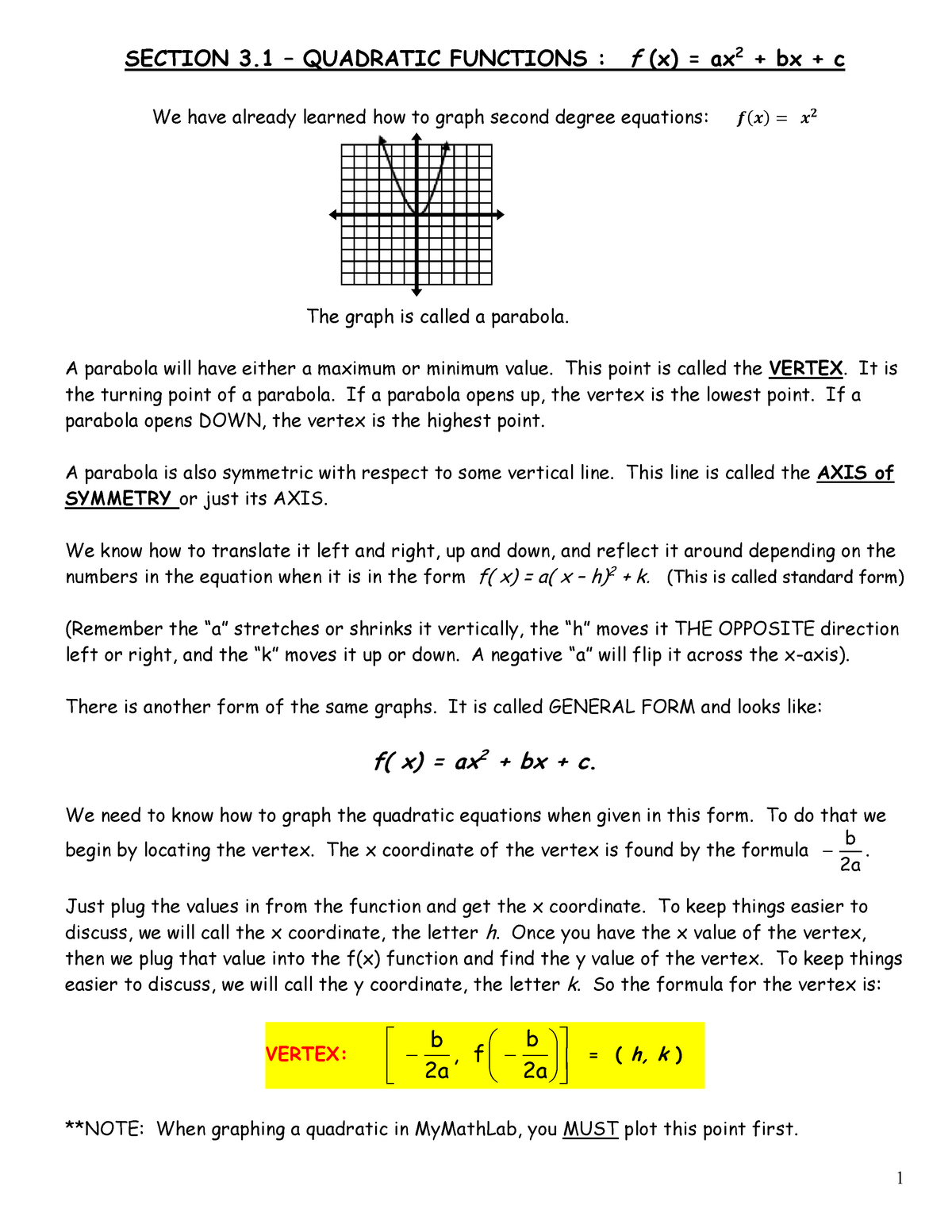



Mac 1105 3 1 Graphing Quadratic Functions Studocu



How To Plot Quadratic When Given The Turning Point And One Of The X Intercepts Socratic



2



Www Thegrangeacademy Co Uk Downloads 6c Quad Cubic Graphs Pdf




Quadratic Functions H Tends To Infinity




Solution Parabola Study Notes Studypool



2




This Professor S Amazing Trick Makes Quadratic Equations Easier The New York Times
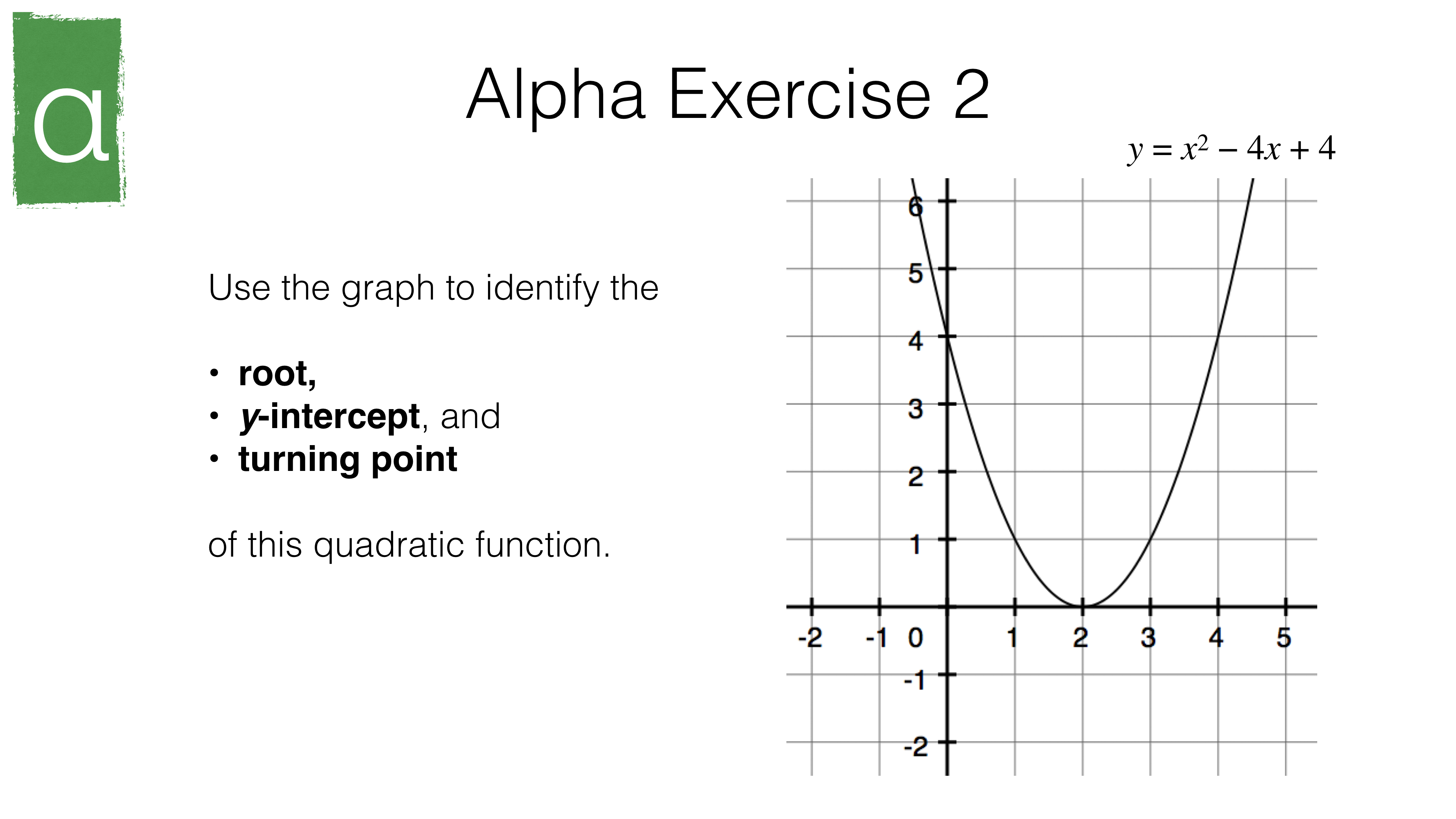



A11a Identifying Roots Intercepts And Turning Points Of Quadratic Functions Graphically Bossmaths Com
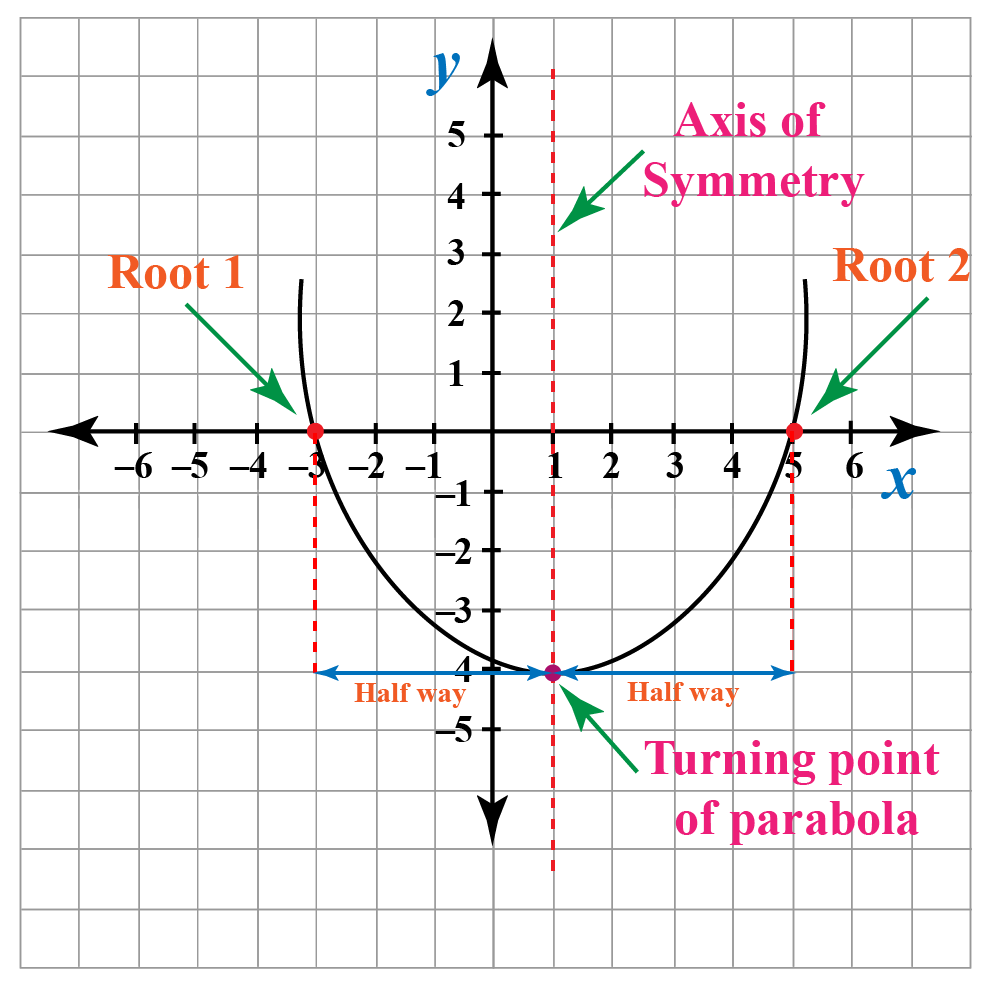



Standard Form In Maths Cuemath
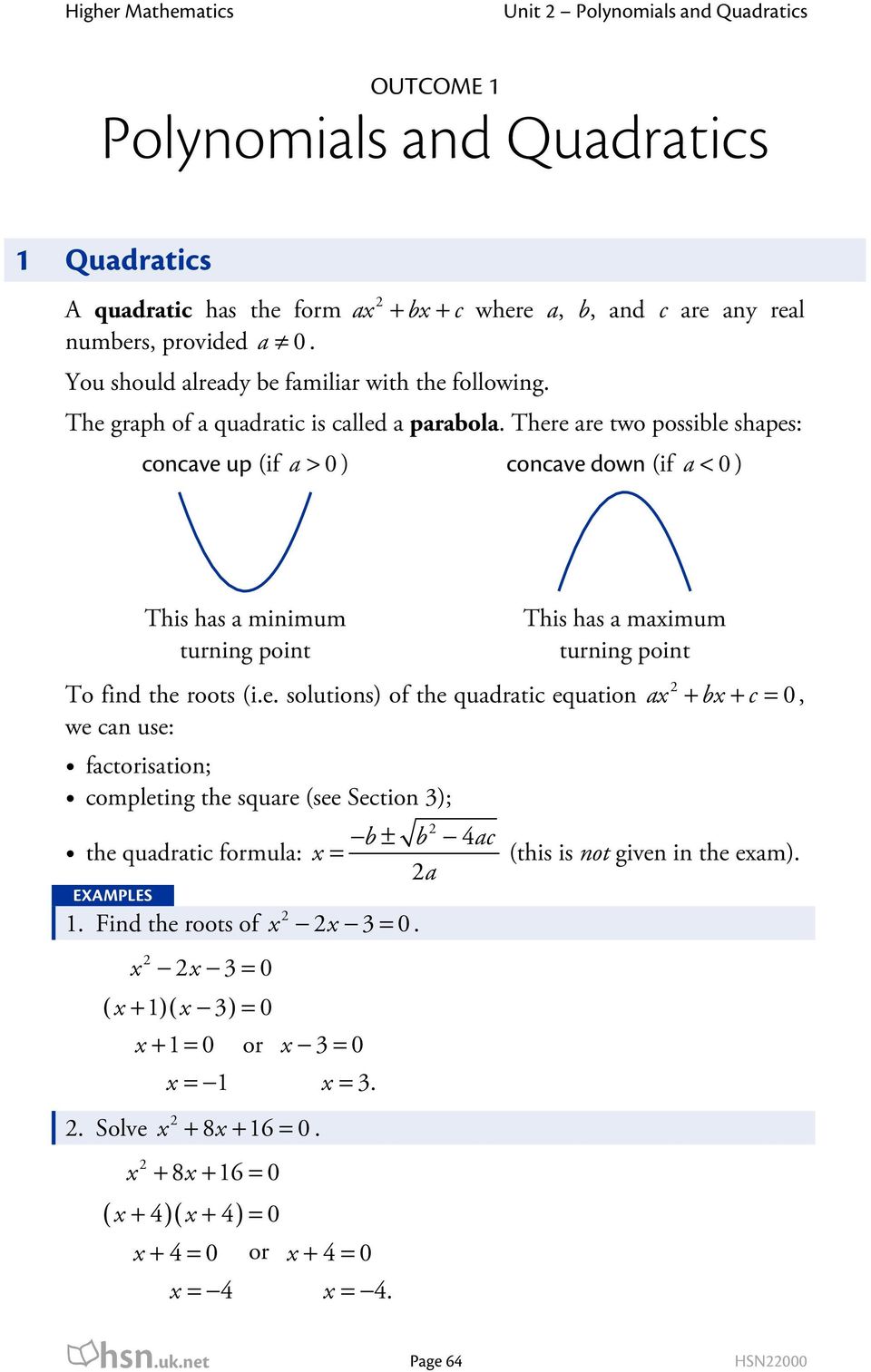



Higher Polynomials And Quadratics 64 Pdf Free Download
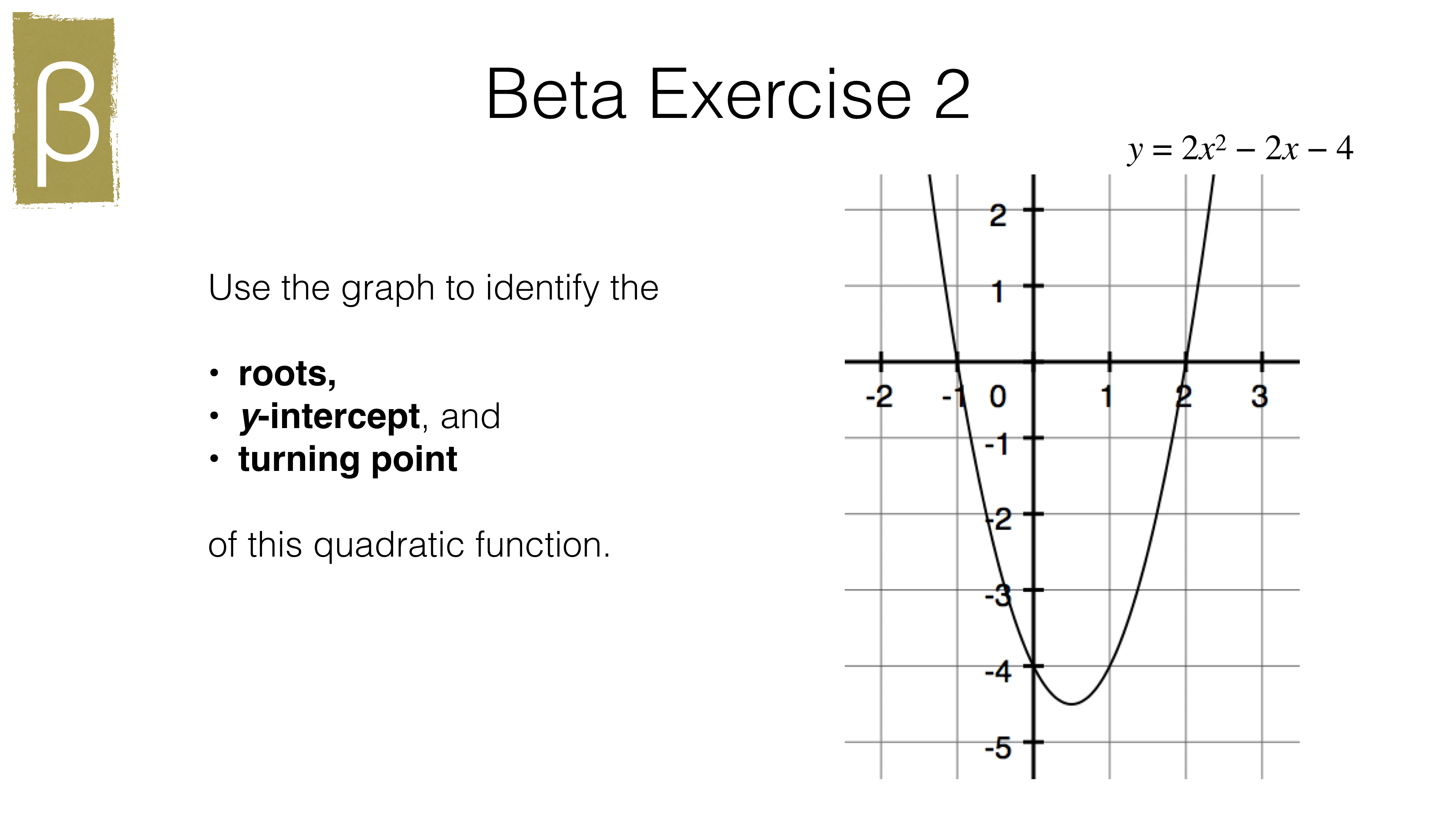



A11a Identifying Roots Intercepts And Turning Points Of Quadratic Functions Graphically Bossmaths Com




Free Response Questions A Quadratic Function Has A Turning Point At 3 8 Selected Values For Brainly Com



2




Higher Polynomials And Quadratics 64 Pdf Free Download



Creating Quadratic Equations Given The Graph Mathbitsnotebook A1 Ccss Math



1




How To Find The Equation Of A Parabola Given The Turning Point



Ib Math Hl Portfolio Parabola Investigation International Baccalaureate Maths Marked By Teachers Com



Olma Org Ourpages Auto 18 10 4 2 5 answers Pdf



Http Www Iroquoiscsd Org Cms Lib Ny Centricity Domain 105 Hw 098 Lesson 13 2 Pdf



2




How To Graph A Parabola 13 Steps With Pictures Wikihow



Sites Levittownschools Com Cbergersen Documents Unit 2 summary answers Pdf




Identify The Axis Of Symmetry Of The Parabola Brainly Com




How To Find The Range Of A Parabola In Vertex Form



Sites Levittownschools Com Cbergersen Documents Unit 2 review sheet answers Pdf



2
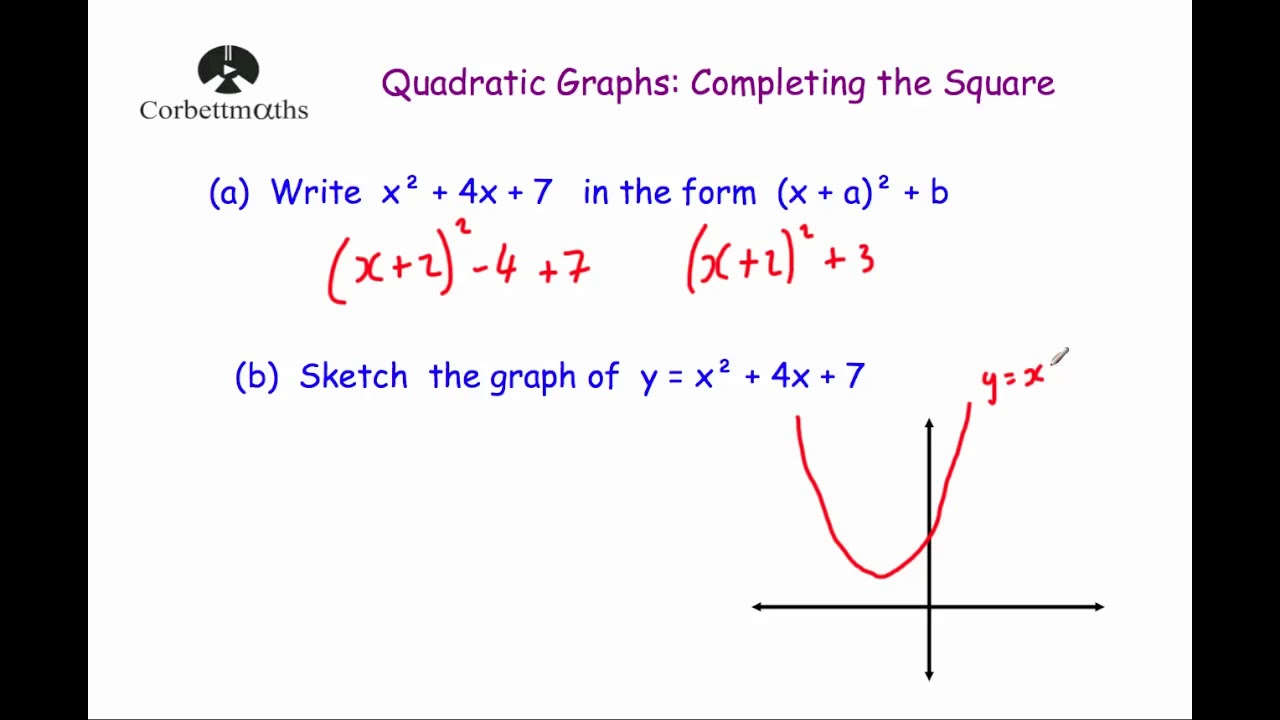



Finding Turning Points Using Completing The Square Youtube
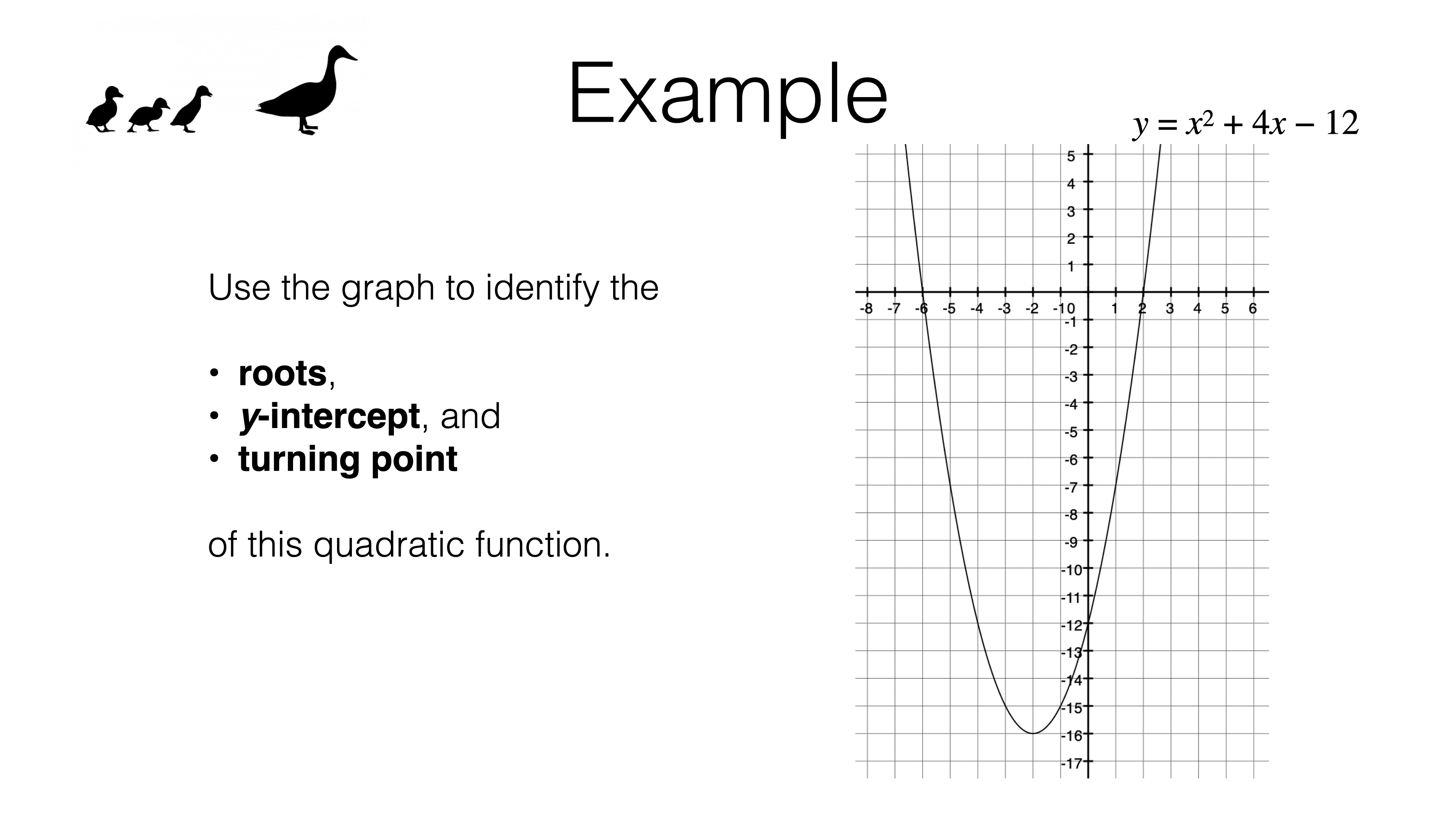



A11a Identifying Roots Intercepts And Turning Points Of Quadratic Functions Graphically Bossmaths Com



Identifying Roots And Turning Points Of Quadratic Functions Gcse Mathematics Edexcel Revision Study Rocket




Parabolas And The Distance Formula Read Calculus Ck 12 Foundation



2



Q Tbn And9gctmj B8gm2opmq Wq6unrehhlmatmfv8ou3nfbf0xpmfzgwmowo Usqp Cau



1
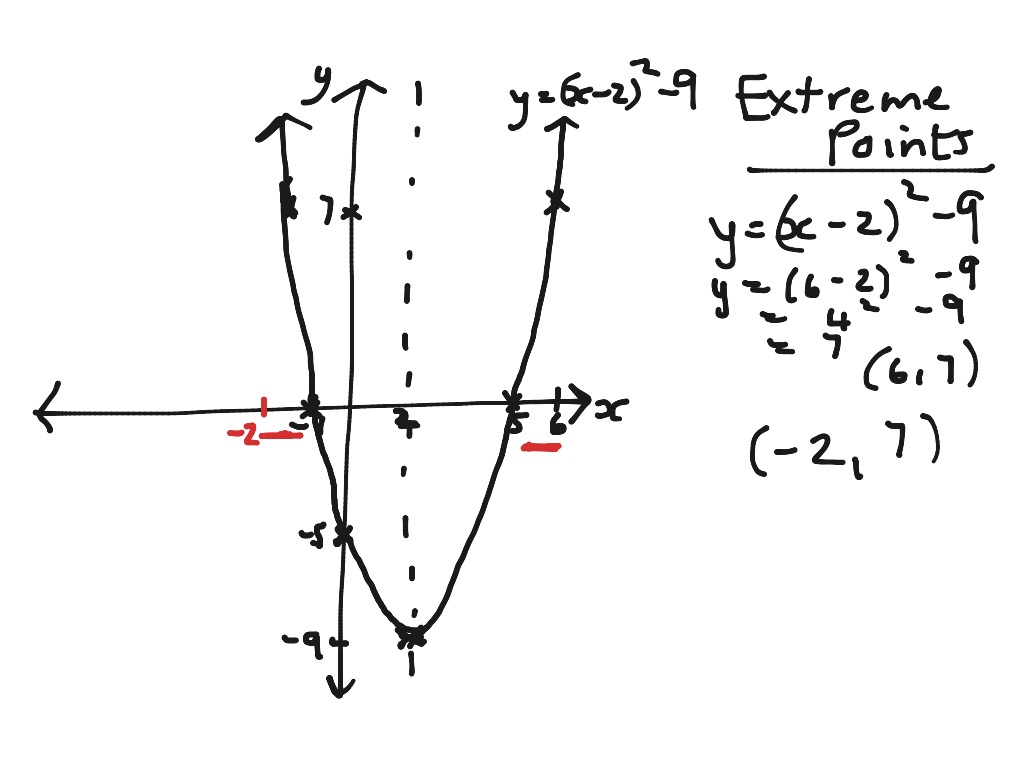



Sketch Quads In Turning Point Form Math Algebra Quadratic Equations Showme



Parabola Equations And Graphs Directrix And Focus And How To Find Roots Of Quadratic Equations Owlcation



Turning Point Formula For Quadratics Youtube



Ib Math Hl Portfolio Parabola Investigation International Baccalaureate Maths Marked By Teachers Com



Answered What Is The Turning Point Of The Bartleby



5 1 The Parabola Mathematics Libretexts



2




Select All Of The Statements That Are True For The Given Parabola Brainly Com
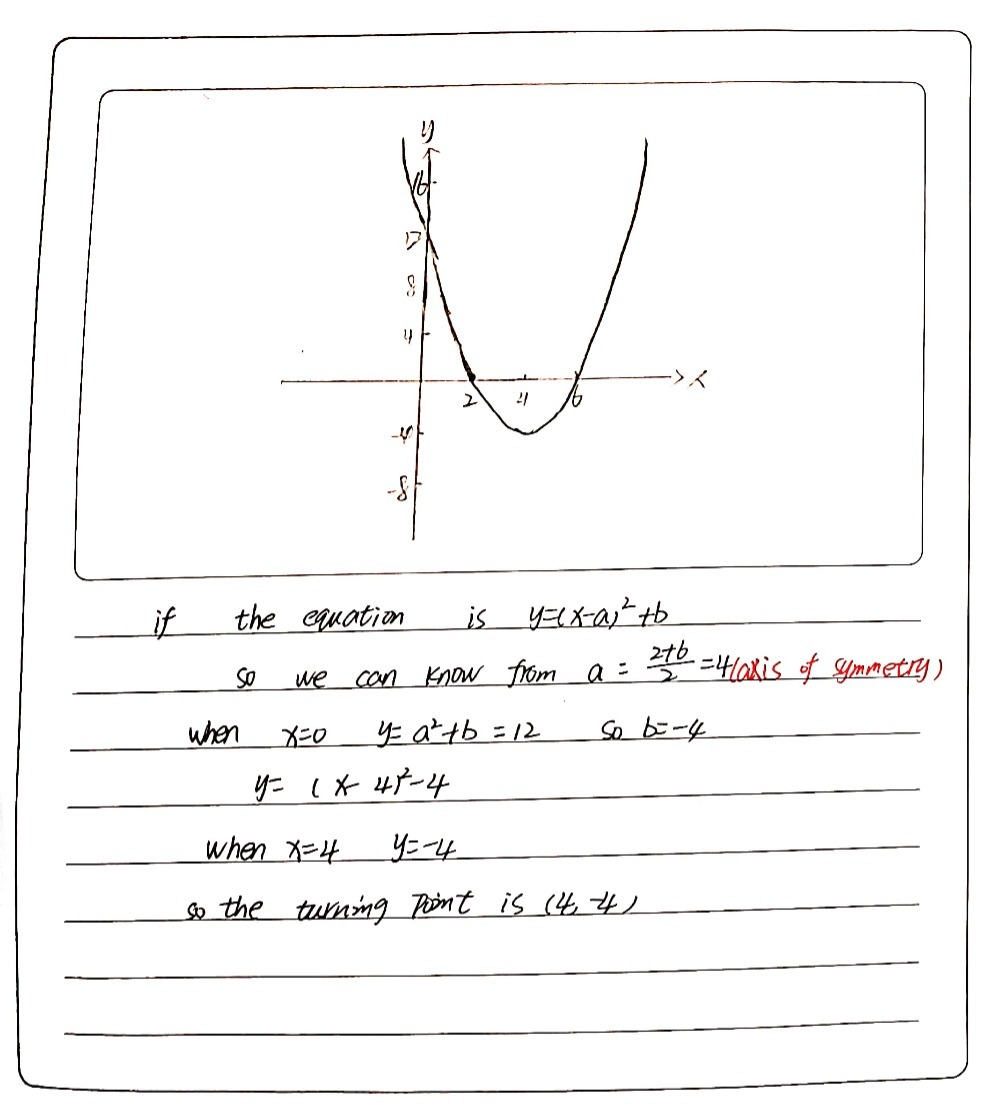



Q 7 Find The Equation And Turning Point Of The Gauthmath
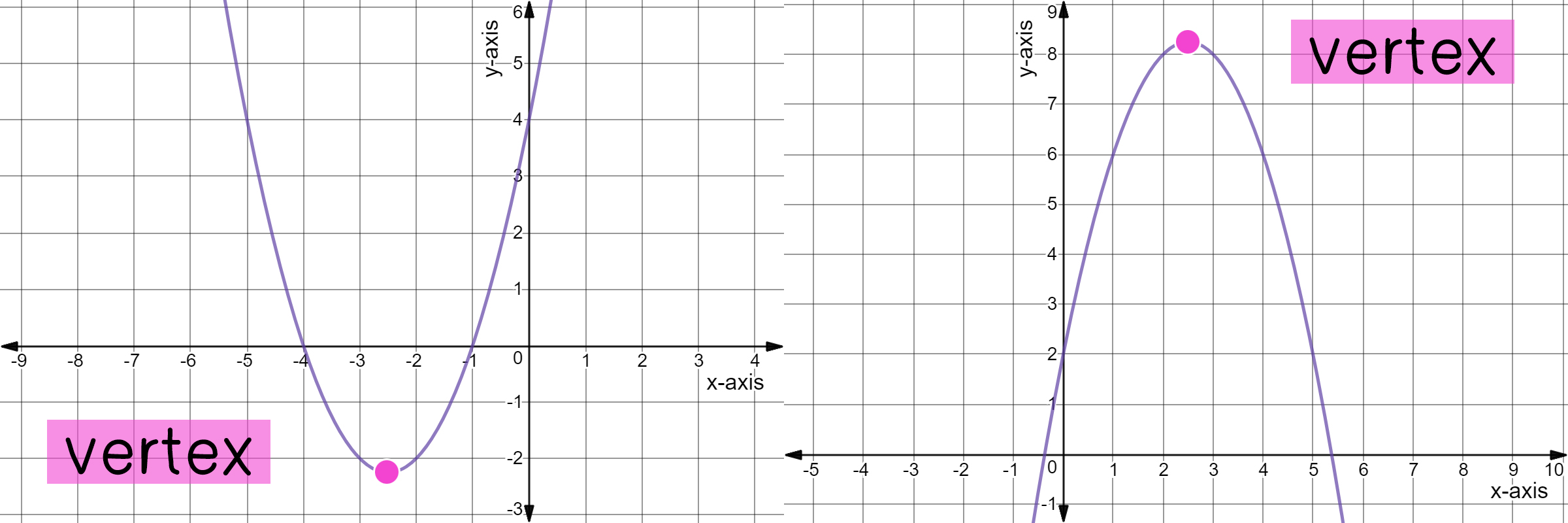



Completing The Square Vertex Form Of A Quadratic Expii




I Understand Finding The Coordinates Of The Turning Chegg Com



2




A Concave Up Parabola Intersects The X Axis At Gauthmath
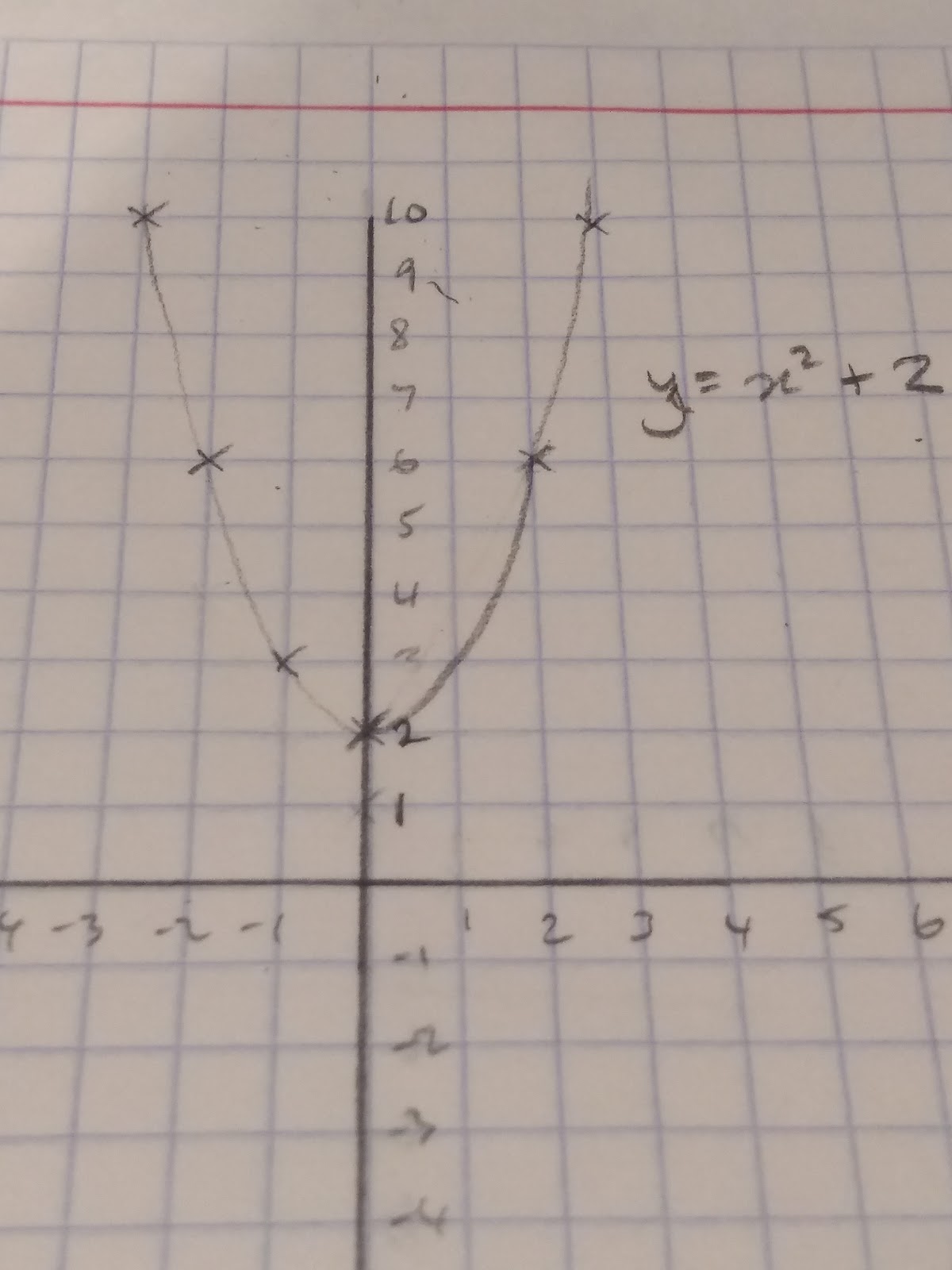



Identifying Roots And Turning Points Of Quadratic Functions Gcse Mathematics Edexcel Revision Study Rocket
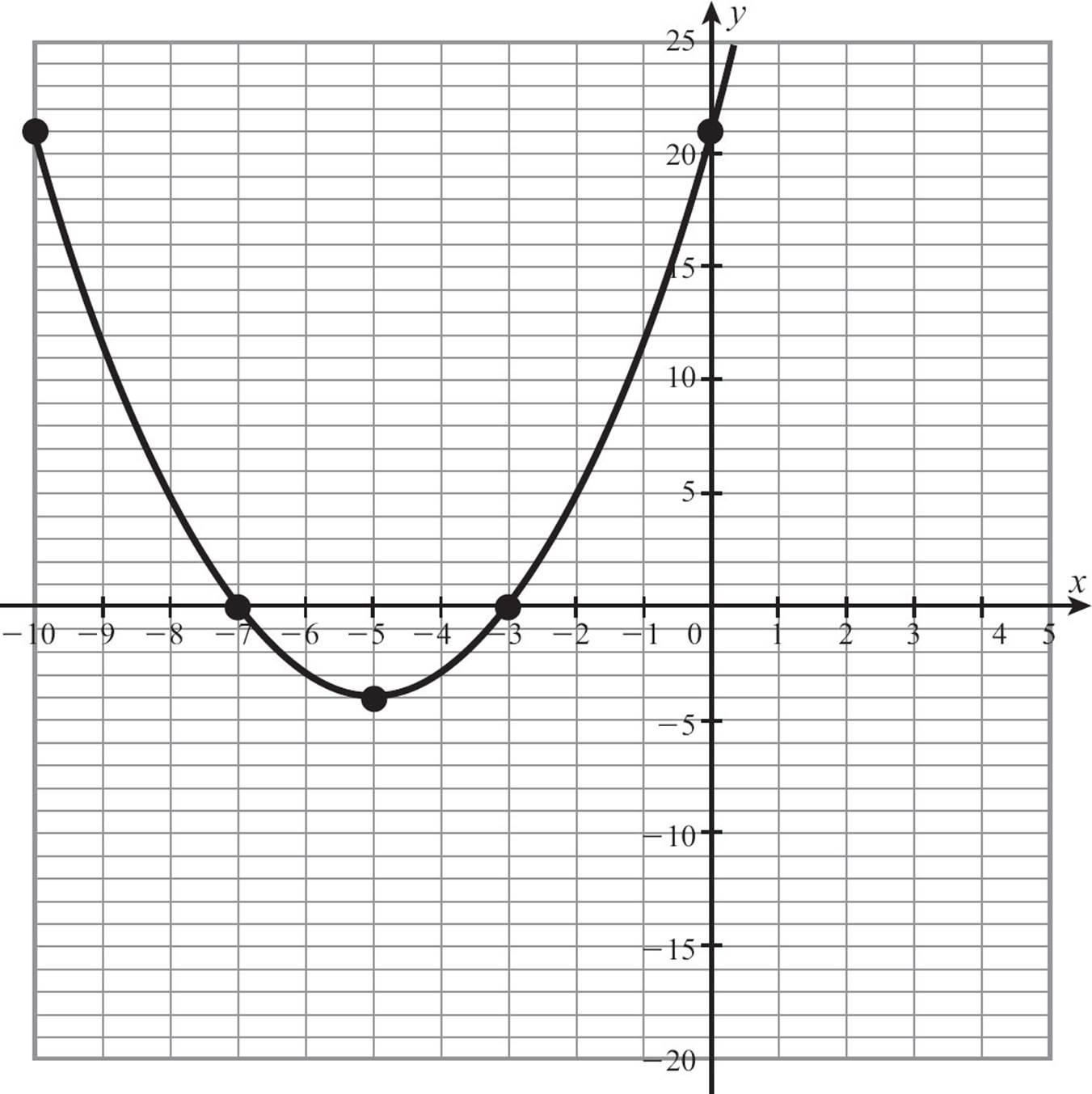



Quadratic Functions Radical Quadratic And Rational Functions Idiot S Guides Algebra I



2




Y X B X C Graph Free Math Help Forum




Yr 12 Maths Textbook Pages 101 150 Flip Pdf Download Fliphtml5




Graphing Quadratic Functions Ppt Download
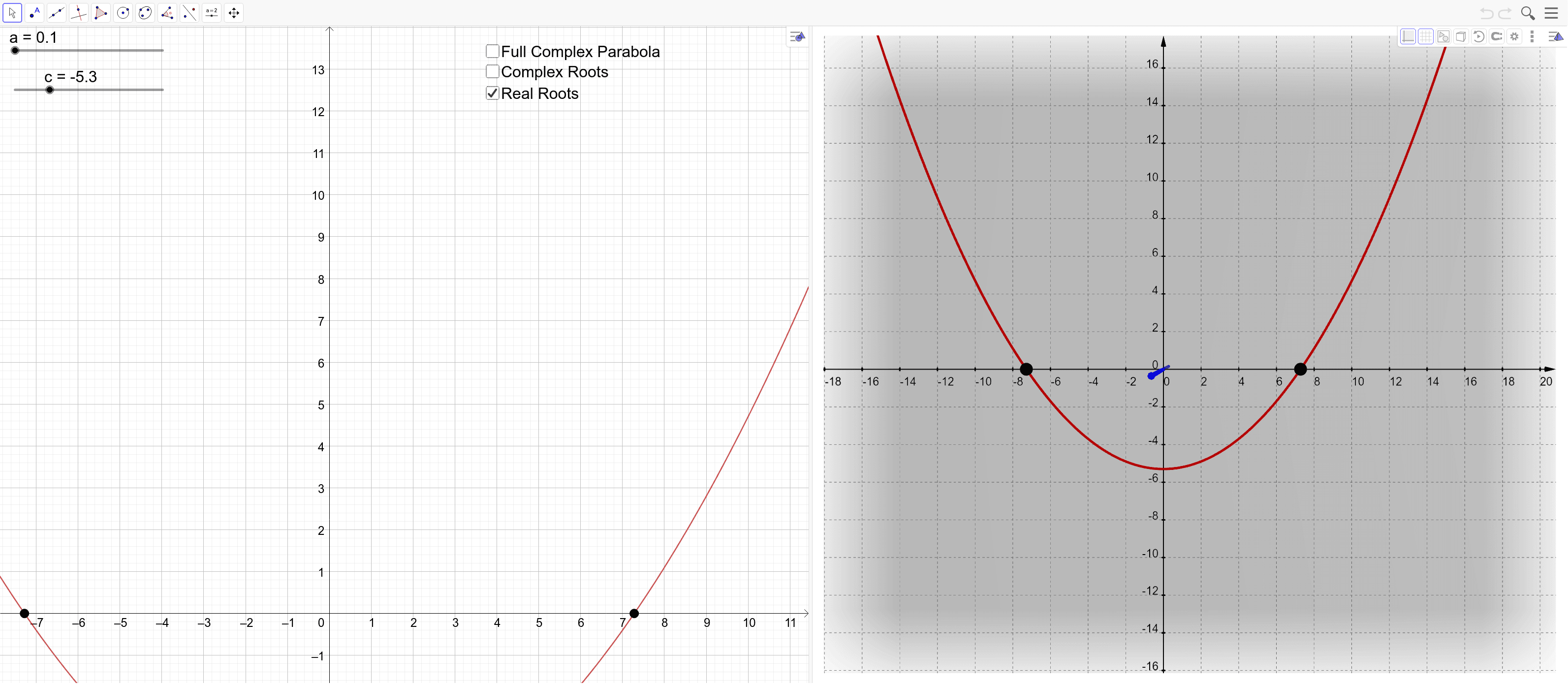



Visualising Complex Roots Of A Parabola Geogebra
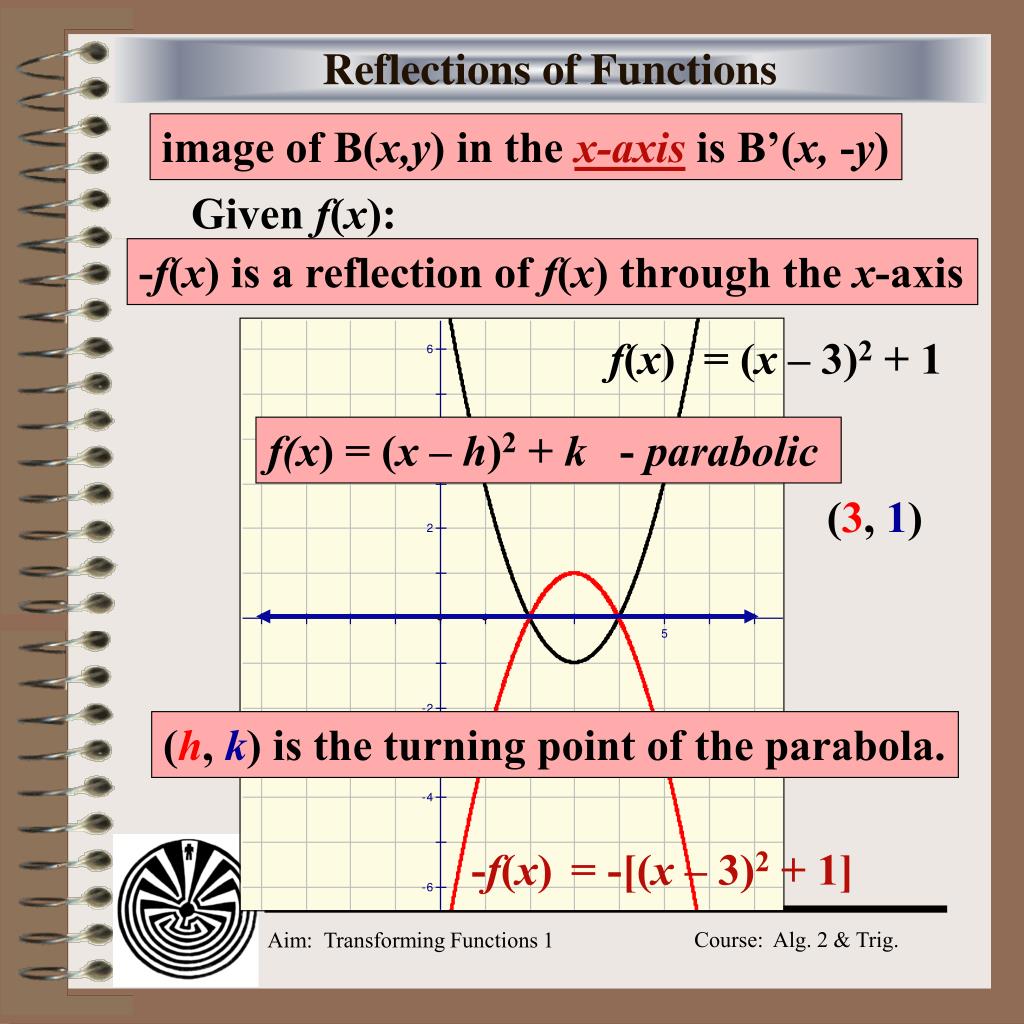



Ppt Aim How Do Transformations Affect The Equations And Graphs Of Functions Powerpoint Presentation Id
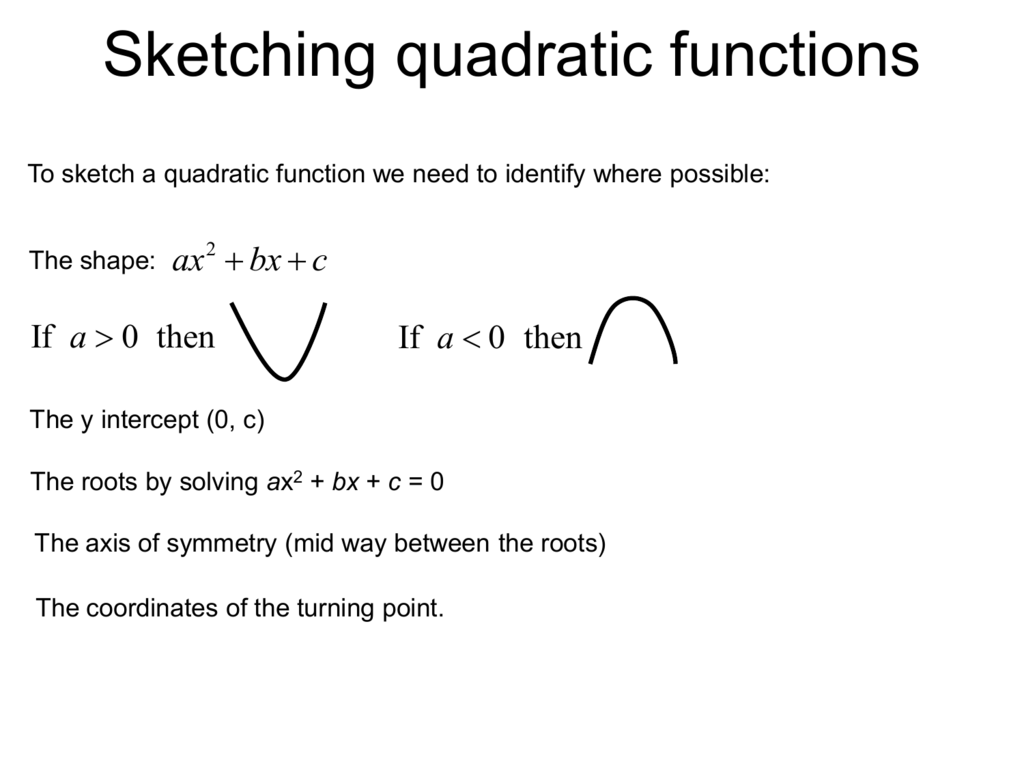



Quadratic Functions




Quadratic Function Wikipedia




How To Analyze A Parabola 6 Steps With Pictures Wikihow



Www Redwoods Edu Portals 121 Intalgtext Chapter5 Section1 Pdf




Higher Polynomials And Quadratics 64 Pdf Free Download




5 1 The Parabola Mathematics Libretexts




How To Draw A Parabola Ppt Download
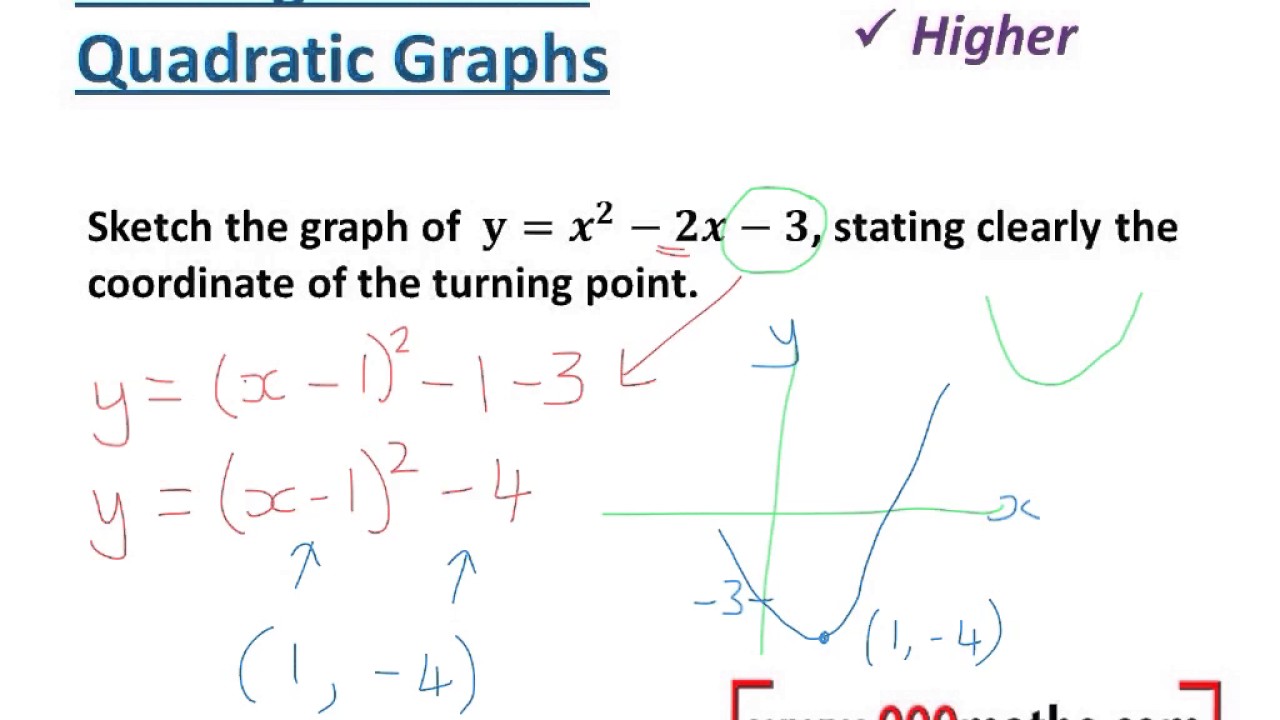



Finding Turning Points Of Quadratics Youtube




Answered What Is The Turning Point Of The Bartleby
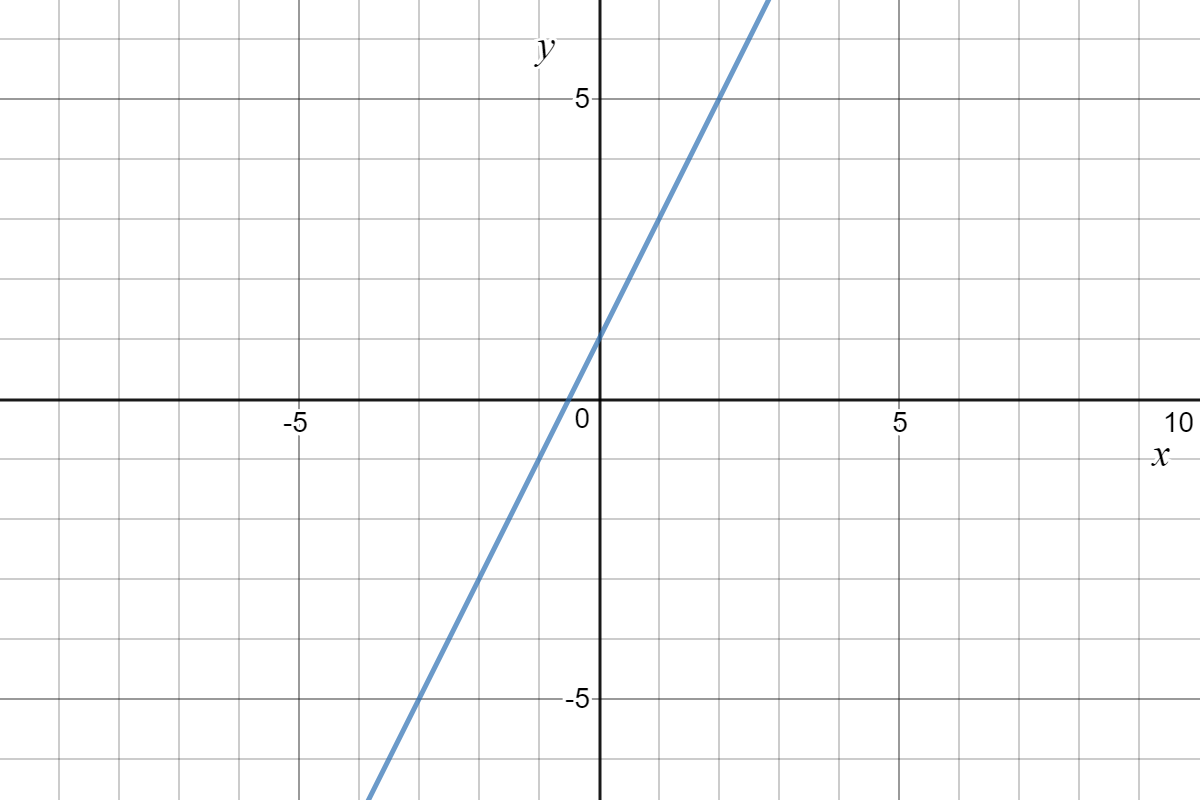



Rate Of Increase Of A Quadratic Function Expii



2
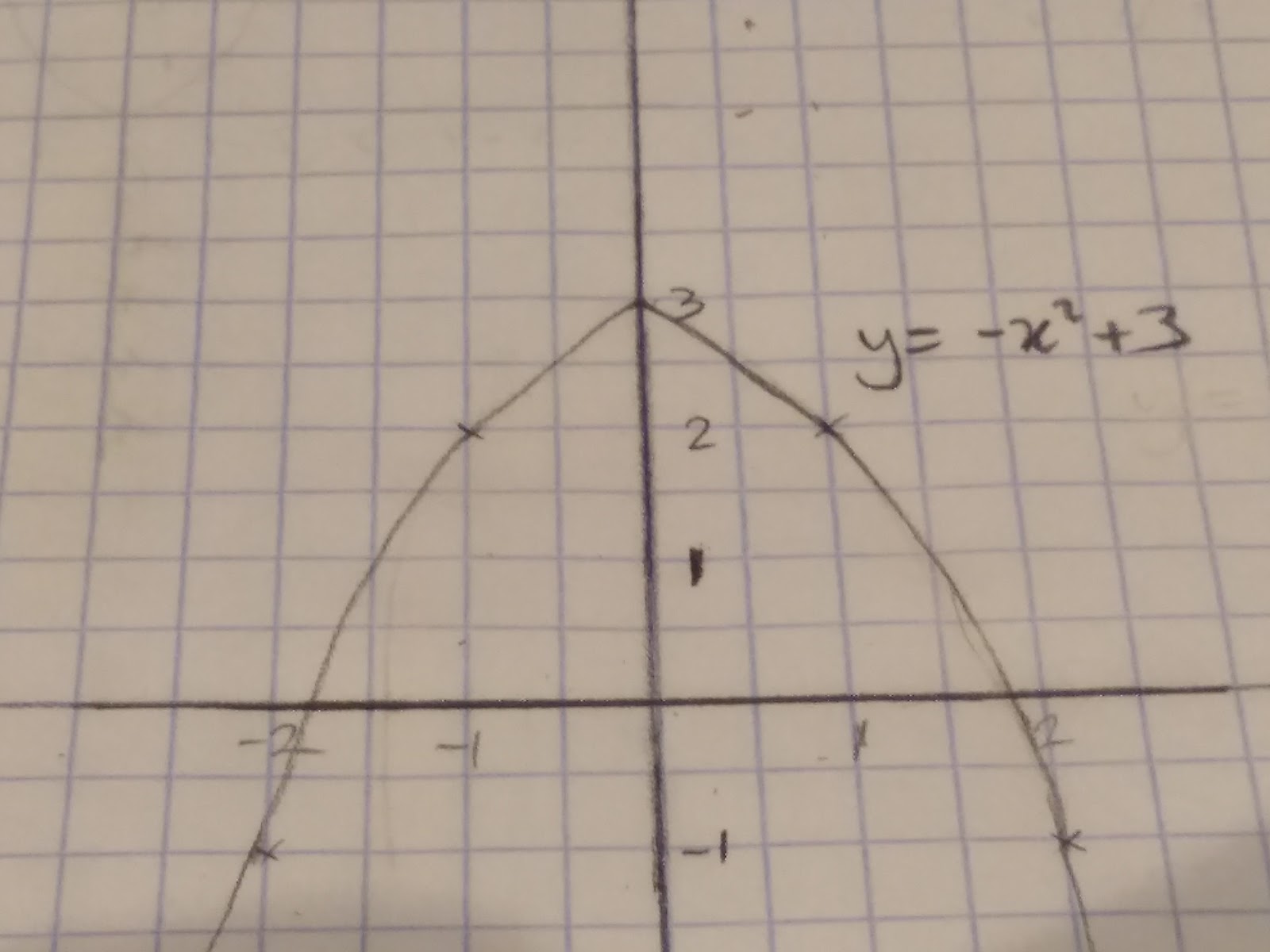



Identifying Roots And Turning Points Of Quadratic Functions Gcse Mathematics Edexcel Revision Study Rocket
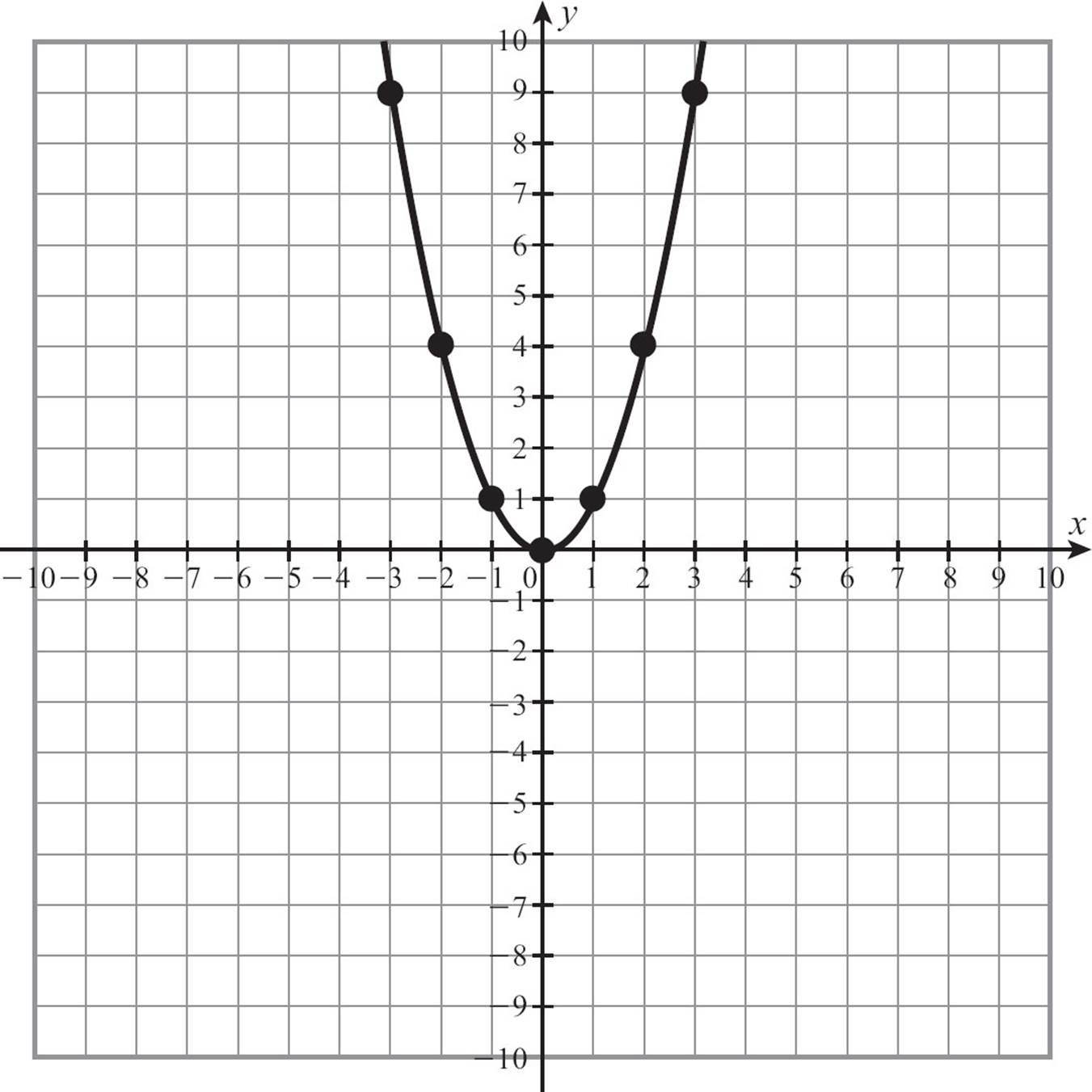



Quadratic Functions Radical Quadratic And Rational Functions Idiot S Guides Algebra I



Http Hawaii Hawaii Edu Math Courses Math026 Notes Lesson55 Pdf




The Sketch Shows Fx Ax2 Bx C And Gx Mx C The Turni Gauthmath




Worksheet Key 11 28 18 9 51 Am 9 2 Parabolas Ppt Download




How To Draw A Parabola Ppt Download




Graph Quadratic Functions In Standard Form Lesson Ppt Download




Differentiation Turning Points Edexcel Igcse Maths Revision Notes



2



Q Tbn And9gcscmkokyxfel78lcn Uouqxabyozx3sh Riaknacehsb3d9 I96 Usqp Cau
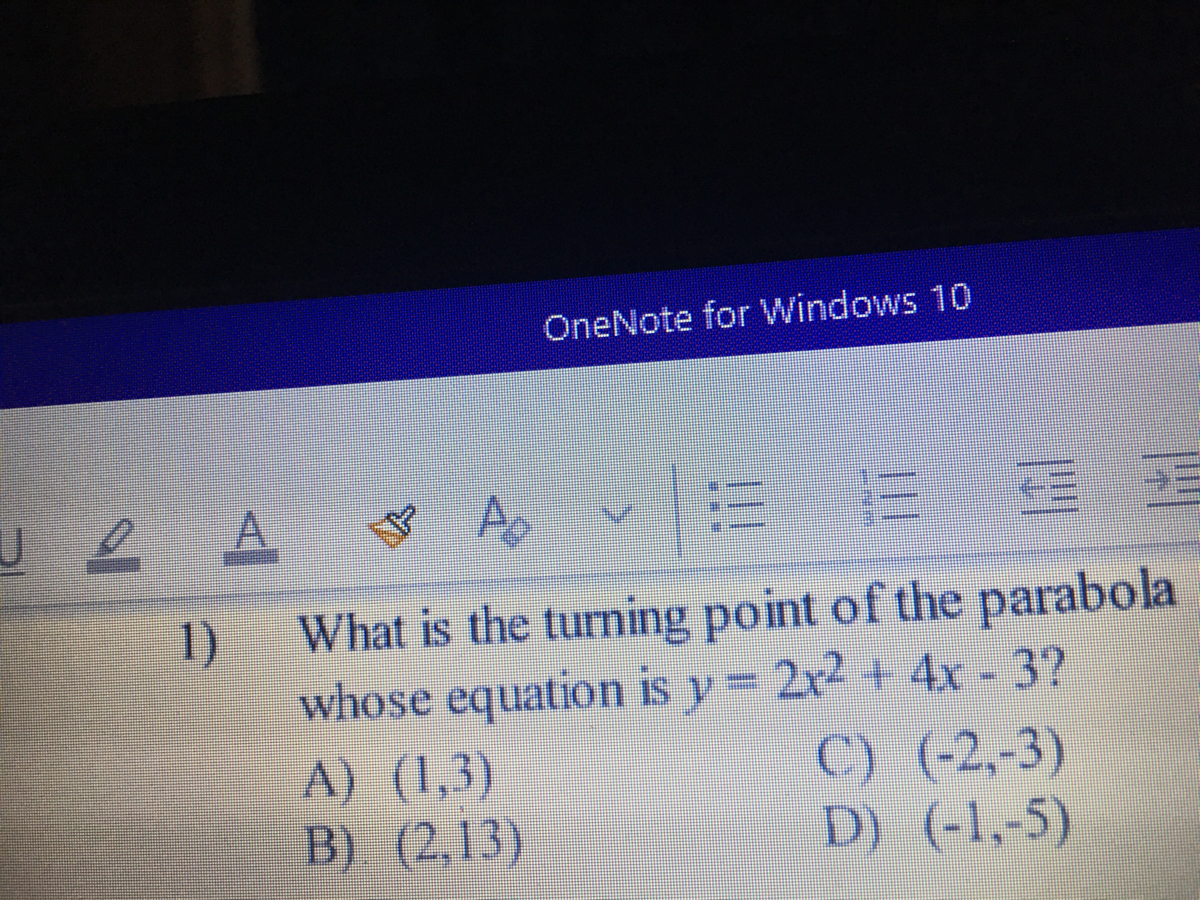



Answered 1 What Is The Turning Point Of The Bartleby



2




5 Functions Straight Lines Parabolas Hyperbolas Exponential Graphs Ppt Download
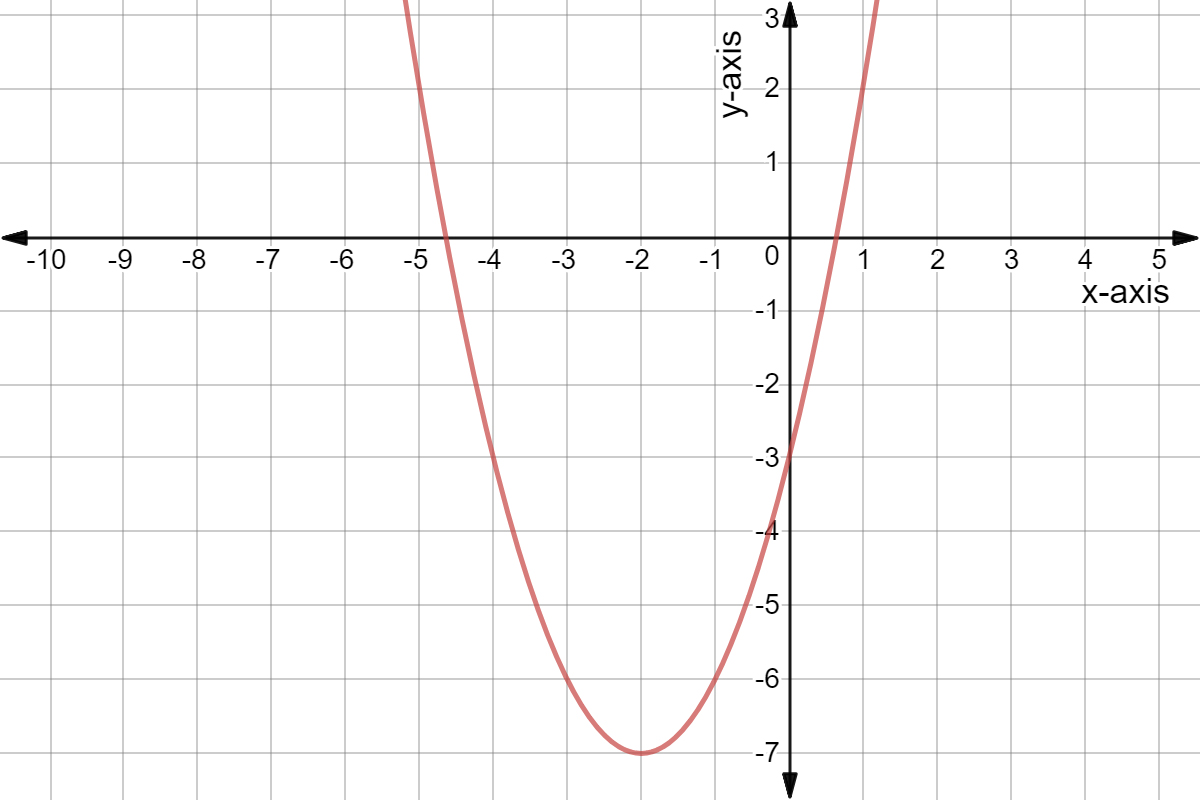



Completing The Square Vertex Form Of A Quadratic Expii


